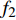Determining heat source parameters based on particle swarm optimization for temperature field simulation of EH40/316L high power laser welding
-
摘要:
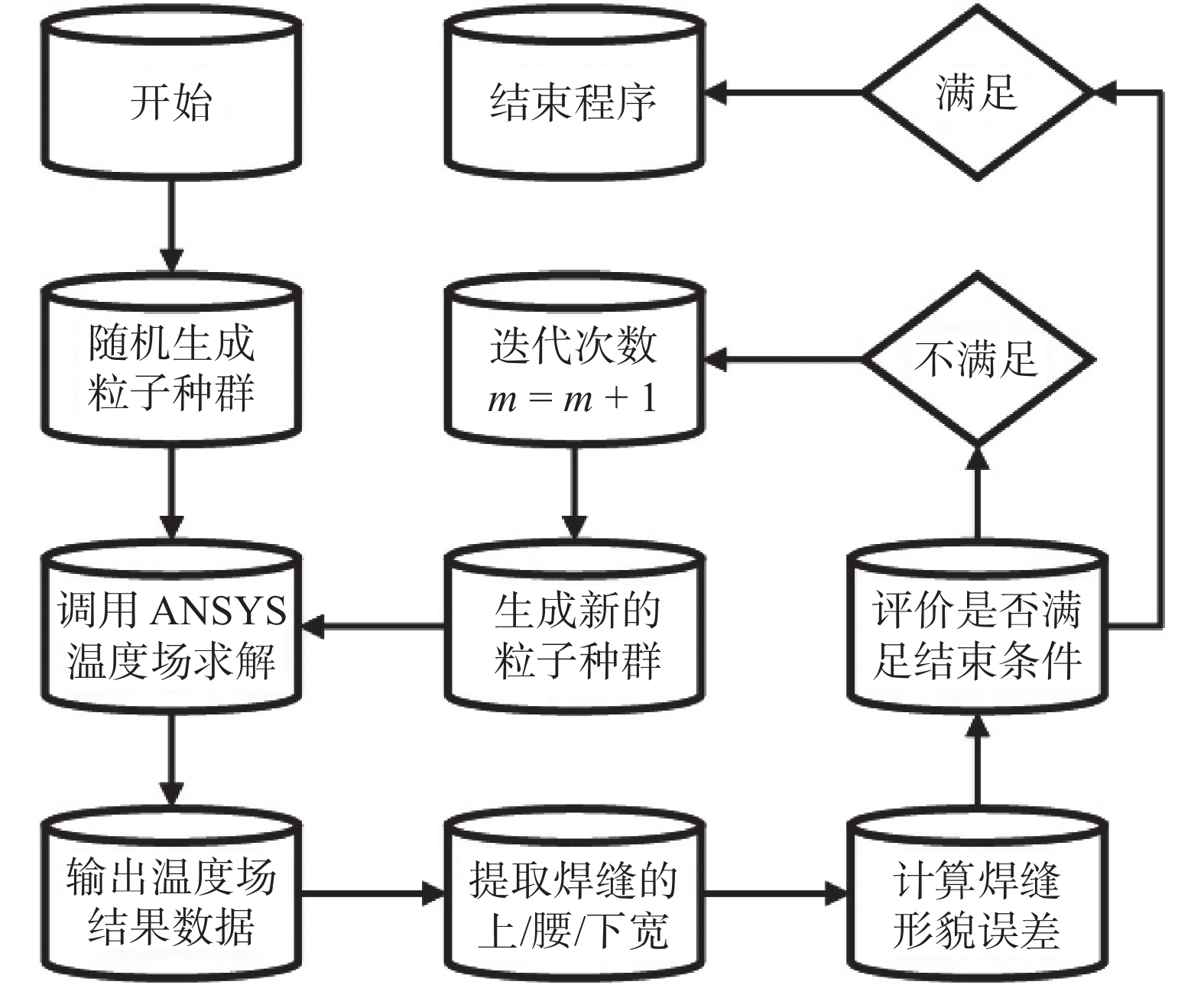
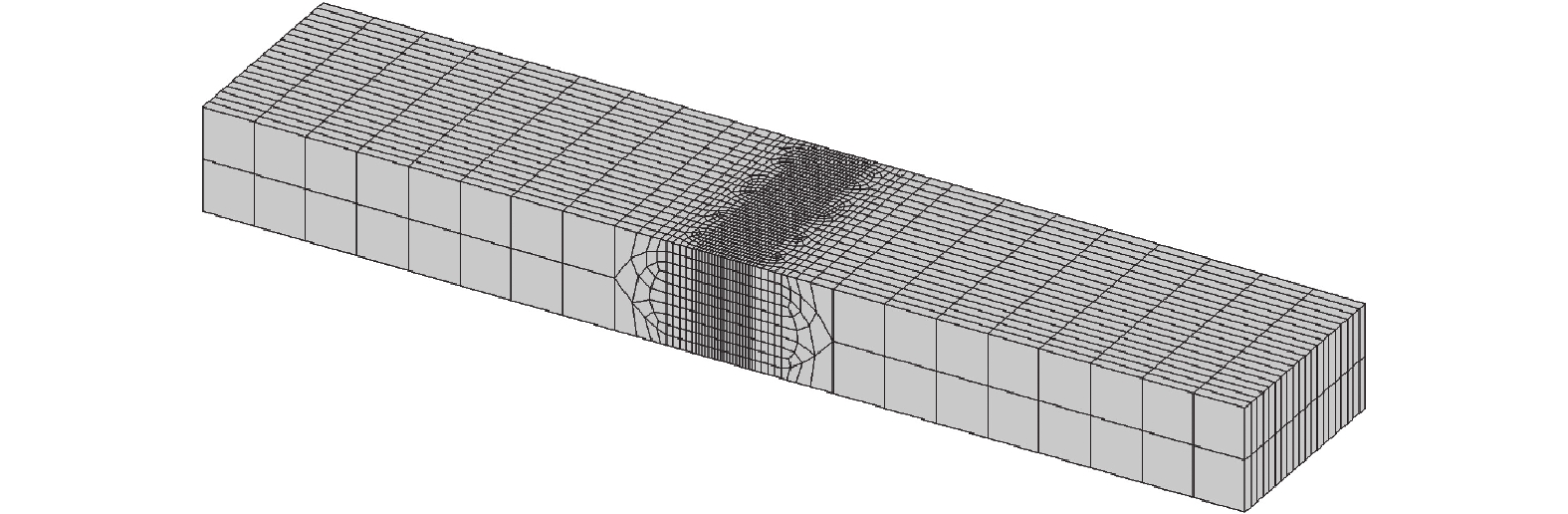
焊接瞬态温度场对焊接残余应力与变形模拟结果具有重要的影响,为提高EH40/316L高功率激光焊接的温度场模拟精度,开发了用于热源模型经验参数优化的智能计算方法.该方法利用MATLAB软件随机生成热源模型的经验参数,调用ANSYS软件执行焊接温度场模拟的APDL命令,并在计算完成后返回结果数据,然后通过借助粒子群算法的群体智能和进化智能的优点,根据预测结果生成新的热源经验参数,进行迭代计算,直至预测结果为最优结果;同时,为提高收敛速度,将有限元几何模型简化为原模型的1/5.基于该方法,开展了10个热源模型经验参数优化案例的计算,并根据优化结果分析了热源模型的参数敏感性.结果表明,该方法实现了温度场的准确预测,其中10个优化案例的平均计算时间为30.3 h,最大、最小和平均预测误差分别为2.84%,2.06%和2.16%;同时,由预测误差和热源经验参数的响应曲面可知,两者之间具有复杂的非线性关系,其数学函数为多谷函数.
Abstract:The welding transient temperature field has an important influence on the simulation results of welding residual stress and deformation. To improve the accuracy of the temperature field simulation in EH40/316L high power laser welding, an intelligent calculation method is developed to optimize the empirical parameters of heat source model. This method uses MATLAB software to randomly generate the empirical parameters, calls ANSYS software to execute the APDL command of the welding temperature field simulation, and returns the result data when the calculation is completed. Then, based on the swarm intelligence and evolutionary intelligence of particle swarm optimization algorithm, new empirical parameters of the heat source are generated according to the prediction results. The iterative calculation is carried out until the prediction results are the optimal results. 10 cases of empirical parameter optimization are performed, and the parameter sensitivity of heat source model is analyzed according to the optimization results. The results show that the average calculation time of the 10 cases is 30.3 hours, and the maximum, minimum and average prediction errors are 2.84%, 2.06% and 2.16% respectively. This indicates a great improvement in the simulation accuracy of welding temperature field. Meanwhile, it can be seen from the response surface of the prediction error and the empirical parameters, that the mathematical function between them is a multi-valley function and has a complex nonlinear relationship.
-
0. 序言
自冲铆(self-piercing riveting, SPR)是一种针对薄板材料的新型机械变形连接技术.连接工艺过程中,作为连接元件的半空心铆钉在高速运行的冲头推动作用下刺入板材,铆钉脚端部产生径向扩张;同时,在底模(通常为弧形凸模或平模)的引导下,被连接材料发生大塑性变形,最终与铆钉形成紧密的机械内锁结构.自冲铆连接具有工艺过程简单、高效、材料适应性广等特点,近年来在航空航天、汽车和家电等轻量化制造领域,成为具有代表性的一种“绿色”机械变形连接技术[1-4].然而,自冲铆连接面对强度较高、塑性相对较差的材料,如7075铝合金板材、铸铝等,连接时形成的机械内锁区容易出现裂纹缺陷,导致结构体的力学性能和抗腐蚀性能下降[5].
针对这一问题,国内外学者提出了不同的解决方案.一方面,通过材料处理的方法,提升基板的延展性,从而改善自冲铆接头的成形质量.Ying等人[6]研究了准静态加载条件下AA7075-T6铝合金板材热自冲铆接的连接质量,通过将铆接温度提高到400 ℃,可以大大提高T-SPR对AA7075-T6铝合金的连接质量.Durandet[7]和Easton[8]等人提出激光-自冲铆接复合工艺,在自冲铆接之前通过激光对镁合金预加热以改善其延展性.Jäckel等人[9]利用底模直径为10 mm、底模深度为1.5 mm的球形模具对6016和7075铝合金进行局部加热并进行自冲铆接,有效地避免了裂纹的产生.另一方面,通过优化底(凹)模结构,降低材料形变过程中局部应力集中.Ma等人[10]探究不同工艺参数对异种板材自冲铆接性能的影响规律,为获得较好的接头强度和避免接头底部开裂可选取较软和较长的铆钉,且模具与铆钉体积比大于1.0的组合.Drossel等人[11]通过优化模具形状且降低模具深度可避免铆接裂纹的产生,但模具深度降低会带来内锁长度过低,并且接头底部也会有裂纹产生.Neuser等人[12]通过减小底模深度,解决了6014铝合金和铸铝在自冲铆接过程中的裂纹问题.Mori等人[13]通过有限元模拟优化了模具的形状,成功将超高强度钢板与铝合金板接合在一起.Li等人[14]研究了模具轮廓对接头质量的影响,具有深角和尖角的模具会使某些材料产生严重的裂纹.
文中提出了一种基于球形底模的自冲铆连接工艺;同时,针对延展性相对较低的7075铝合金,应用响应面法建立自冲铆接头失效载荷和能量吸收值的多元回归模型,以探究自冲铆成形过程中各影响因素及其交互作用对力学性能的影响规律.
1. 试件材料与响应面设计
图1为所选用的自冲铆接试验设备,铆接对象为高强度7075-T6铝合金,铆钉管腿内径为3.5 mm,外径为5.3 mm,硬度均为H4(330 HV)的半空心铆钉.预试验分析后发现使用传统底膜均有裂纹产生(图2),选用底模深度为1 mm,直径为10 mm的球形模具没有裂纹产生,其实物图、截面尺寸和成形接头底部如图3所示. 采用不同底模和相同铆接参数成形的接头强度数据对比如图4所示. 7075-T6铝合金板材的力学性能如表1所示.被连接板材的厚度为1.5 mm + 2 mm,试件尺寸均为110 mm × 20 mm,采用单搭铆接,搭接区域为20 mm × 20 mm,试件几何形状和尺寸如图5所示.
表 1 7075-T6铝合金力学性能参数Table 1. Mechanical property parameters of 7075-T6 aluminium alloy材料 弹性模量E/GPa 屈服强度ReL/MPa 抗拉强度Rm/MPa 断后伸长率A(%) 7075-T6 71 325 430 13 BBD法是通过试验设计并借助试验得到一定的试验数据,采用多元回归方程建立响应值与输入变量之间的函数关系式,通过分析变量与响应值之间的变化趋势,从而进行工艺调控与参数预测. 文中分别以自冲铆接头的失效载荷、能量吸收值为响应值,以铆钉长度、冲头速度和冲头行程为影响因素,利用Box-Behnken试验设计中的三因素三水平试验设计方法,试验因素水平设计如表2所示,利用Design Expert10.0.1软件得到表3中的BBD试验组合.为减小误差,每组试件制备四个,取其中任意一个进行实际剖切,测量内锁长度、残余底厚等机械内锁结构尺寸,其余三个在MTSlandmark100材料试验机上进行拉伸速率为5 mm/min的拉伸-剪切试验(图6),以探究各工艺参数对接头静力学性能的影响.考虑到试件两端未在同一水平面上,因此在两端夹持与试件等厚的垫片来消除扭矩.
表 2 三因素三水平设计表Table 2. Design table of three factors and levels影响因素 因子水平 −1 0 1 铆钉长度L/mm 5 5.5 6 冲头速度V/(mm·s−1) 20 40 60 冲头行程D/mm 64 65 66 表 3 自冲铆接头拉伸试验结果Table 3. Tensile test results of self-piercing riveting joints组数 铆钉长度L/mm 冲头速度V/(mm·s−1) 冲头行程D/mm 失效载荷F/N 能量吸收值W/J 1 5 40 66 4667.614 4.673 2 5.5 40 65 5286.160 11.845 3 6 40 64 5259.394 19.826 4 5 20 65 4395.852 4.320 5 5.5 40 65 5386.505 11.941 6 6 40 66 5232.434 16.000 7 5 60 65 4196.894 3.955 8 5.5 20 64 4600.116 11.527 9 5.5 20 66 5381.428 10.946 10 5.5 40 65 5218.264 10.174 11 5.5 60 66 5390.007 14.960 12 5.5 60 64 4895.333 14.012 13 6 60 65 5064.895 17.496 14 5 40 64 4376.358 5.617 15 6 20 65 5353.075 20.230 2. 试验结果及分析
2.1 响应面模型的建立
通过对试验数据采用多元二次回归方程拟合,剔除显著性较低项,优化后得到接头的失效载荷和能量吸收值的响应面回归模型如式(1)、式(2)所示,并通过方差分析对模型显著性进行检验,$P$值反映模型中各因素的显著性,当$P < 0.01$时,认为该因素高度显著;当$0.01 < P < 0.05$时,则该因素显著,并根据各因素$P$值进行模型优化.
失效载荷模型:
$$ \begin{split} {y_1} = &- 122\;626 + 27\;077.652\;69{x_1} + 279.406\;62{x_2} +\\& 1\;210.958\;75{x_3} - 2.230\;55{x_1}{x_2} - 159.110\;00{x_1}{x_3} - \\& 3.582\;97{x_2}{x_3} - 1\;438.910\;15x_1^2 - 0.442\;39x_2^2 \end{split} $$ (1) 能量吸收值模型:
$$\begin{split} {y_2} =& - 639.685\;84 + 139.968\;68{x_1} + 0.346\;99{x_2} + \\& 7.375\;13{x_3} - 0.059\;225{x_1}{x_2} - 1.441\;00{x_1}{x_3} - \\& 2.744\;36x_1^2 \end{split} $$ (2) 式中:${y_1}$、${y_2}$为响应值,分别表示失效载荷和能量吸收值,${x_1}$、${x_2}$、${x_3}$为输入变量,分别表示铆钉长度、冲头速度和冲头行程.其中失效载荷是评价构件抵抗外力破坏能力最直接的手段,也是评价自冲铆接头质量的重要衡量指标.由表4单因素方差分析可知,失效载荷和能量吸收值均受铆钉长度的影响最为显著,冲头行程次之,冲头速度影响最弱.各组铆接接头对应的载荷—位移曲线和截面如图7、图8所示.
表 4 方差分析表Table 4. Analysis of variance table响应值 项目 平方和 自由度 均方误差 F值 P值 显著性 失效载荷 模型 2.254 × 106 8 2.817 × 105 6.83 0.0154 显著 $ {x}_{1} $ 1.339 × 106 1 1.339 × 106 32.49 0.0013 $ {x}_{2} $ 4.202 × 103 1 4.202 × 103 0.10 0.7603 $ {x}_{3} $ 2.966 × 105 1 2.966 × 105 7.19 0.0364 $ {x}_{1}{x}_{2} $ 1.990 × 103 1 1.990 × 103 0.05 0.8334 $ {x}_{1}{x}_{3} $ 2.532 × 104 1 2.532 × 104 0.61 0.4630 $ {x}_{2}{x}_{3} $ 2.054 × 104 1 2.054 × 104 0.50 0.5067 $ {x}_{1}^{2} $ 4.806 × 105 1 4.806 × 105 11.66 0.0142 $ {x}_{2}^{2} $ 1.163 × 105 1 1.163 × 105 2.82 0.1440 失拟项 2.330 × 105 4 5.825 × 104 8.13 0.1125 不显著 能量吸收值 模型 387.05 6 64.51 22.09 0.0001 显著 $ {x}_{1} $ 377.95 1 377.95 129.42 <0.0001 $ {x}_{2} $ 1.44 1 1.44 0.49 0.5017 $ {x}_{3} $ 2.42 1 2.42 0.83 0.3890 $ {x}_{1}{x}_{2} $ 1.40 1 1.40 0.48 0.5078 $ {x}_{1}{x}_{3} $ 2.08 1 2.08 0.71 0.4236 $ {x}_{1}^{2} $ 1.76 1 1.76 0.60 0.4602 失拟项 21.39 6 3.56 3.61 0.2327 不显著 为验证所建立响应面模型的准确性,对回归模型进行试验验证,在所设定的铆接工艺参数范围内随机选取两组参数进行试验设计,并将设计的铆接工艺参数分别代入失效载荷和能量吸收值回归模型计算公式中.表5为输入参数下试验结果的均值、模型预测值以及误差率.第Ⅰ组的失效载荷和能量吸收值试验均值与模型预测值误差分别为2.45%、9.51%;第Ⅱ组的失效载荷和能量吸收值试验均值与模型预测值误差分别为4.64%、7.45%,误差均在合理范围内,表明所建立的回归模型具有较高的可靠性,可据此进行基于球形底模的铝合金自冲铆接工艺调控及参数预测.
表 5 回归模型验证Table 5. Regression model verification组号 铆钉长度
L/mm冲头速度
V/(mm·s−1)冲头行程
D/mm失效载荷试验均值
Fx/N失效载荷预测值
Fy/N误差
ef (%)能量吸收值试验均值
Wx/J能量吸收值预测值
Wy/J误差
ew (%)Ⅰ 5.5 30 65 5108.727 5233.767 2.45 13.248 11.988 9.51 Ⅱ 5.5 50 64 4830.033 5054.148 4.64 14.008 12.964 7.45 2.2 铆接参数对失效载荷和能量吸收值的影响
为探究各单因素对失效载荷的影响规律,可把其余两个因素固定在中间值.如图9(a)所示,铆钉长度的增加,接头的失效载荷会先增大后减小,当铆钉长度达到5.7 mm左右,失效载荷达到最大值.冲头速度对失效载荷的影响呈现先上升后下降的变化规律,变化幅度不大,在40 mm/s左右时,失效载荷达到最大值.不同冲头行程下的失效载荷变化趋势呈线性增加.交互作用方差分析中Px1x3(0.4630)<Px2x3(0.5067)<Px1x2(0.8334),即铆钉长度与冲头行程的交互作用对失效载荷的影响最显著,冲头速度与冲头行程的交互作用影响次之,而铆钉长度和冲头速度的交互作用影响最弱. 图9(b)、(c)对铆钉长度和冲头行程的交互作用分析,可以看出左侧等高线比右侧稠密,且上半部分等高线比下半部分稠密.表明铆钉长度和冲头行程对失效载荷的影响都比较明显,当冲头行程处于设定范围内的最大值,铆钉长度位于5.7 mm左右时,失效载荷可达到峰值.这是因为较长的铆钉搭配合适的冲头行程使铆钉下行越充分,更容易促进铆钉与板材之间的塑性流动,致使较长的铆钉可以在上下板之间充分扩张,形成更加显著的机械内锁结构.
能量吸收值大小是评价构件的缓冲吸震能力,能量吸收值愈大,构件抵抗弹塑性变形的能力愈大. 铆接参数对能量吸收值的影响见图10.由图10(a)可以看出,铆钉长度对能量吸收值的影响最为显著,且随着铆钉长度的不断增大,能量吸收值呈现线性增加.冲头速度的变化对能量吸收值的影响较小,其变化趋势近似为一条直线.随着冲头行程的增加能量吸收值逐渐减小,但总体变化幅度不大.方差分析结果Px1x3(0.4236)<Px1x2(0.5078),冲头速度为40 mm/s时,铆钉长度与冲头行程的交互作用对能量吸收值的影响规律如图10(b)、(c)所示,可以看到能量吸收值会随着铆钉长度的增大而显著增加,且能量吸收值的最高点出现在铆钉长度为最大值,冲头行程为最小值处.结合等高线图可知,冲头行程的改变对能量吸收值的影响并不显著,但是,随着铆钉长度的增大,能量吸收值呈线性增加,这是由于铆钉长度的增大增加了接头的内锁长度,接头抵抗变形的能力也随之增大.因此为获得较高的能量吸收值,应优先考虑合适的铆钉长度.
3. 工艺参数优化
基于响应面模型,运用NSGA-II遗传算法对目标函数进行迭代优化.设置交叉概率80%,变异概率20%,种群规模为50,迭代次数为200.结合试验结果可知,由于铆钉尺寸已标准化,选取5.5 mm长的铆钉时自冲铆接头有较大的内锁长度和接头强度,故铆钉长度选取5.5 mm.为获得较优的失效载荷和能量吸收值,建立多目标优化的目标函数和约束条件为
$$ \begin{gathered} g(x) = min{\text{ }}[{y_1},{y_2}] \\ {\text{ }}s.t.\left\{ {\begin{array}{*{20}{c}} {{x_1} = 5.5} \\ {20 \leqslant {x_2} \leqslant 60} \\ {64 \leqslant {x_3} \leqslant 66} \end{array}} \right. \\ \end{gathered} $$ (3) 式中:${y_1}$、${y_2}$分别为失效载荷和能量吸收值,${x_1}$、${x_2}$、${x_3}$分别对应为铆钉长度、冲头速度和冲头行程.
经运算后得到了目标函数的Pareto最优解解集,Pareto最优解解集是指在可行决策空间内,一组各目标都较优的参数解的集合[15].图11为Pareto前沿图,每个点对应了一个Pareto最优解.最优参数解的选取应使接头有较高的失效载荷和能量吸收值.因此选择图11中的M点作为最优工艺参数:铆钉长度5.5 mm,冲头速度36.6 m/s,冲头行程66 mm.对应的失效载荷为5438.820 N,能量吸收值为11.662 J, 并对最优工艺参数进行试验验证.失效载荷和能量吸收值的试验值分别为5191.482 N、12.376 J.试验值与模型预测值误差分别为4.76%、5.77%.通过工艺参数优化,不仅抑制自冲铆接头机械内锁区裂纹的产生,而且在保证连接质量的情况下获得较高的失效载荷和能量吸收值.
4. 结论
(1) 对于低延展性铝合金薄板材料,采用球形底模在保证接头强度下可以抑制接头机械内锁区裂纹的产生.
(2) 采用BBD响应面法,建立基于球形底模的铝合金自冲铆接工艺参数多元非线性回归模型.实际应用中可以选用铆钉长度、冲头速度以及冲头行程实现自冲铆接工艺参数优化和接头质量调控.
(3) 铆钉长度对失效载荷和能量吸收值的影响最为显著,冲头行程影响次之,冲头速度影响最弱;多因素交互影响中,铆钉长度和冲头行程的交互作用对失效载荷和能量吸收值的影响最大,应优先进行铆钉长度和冲头行程的调控.
-
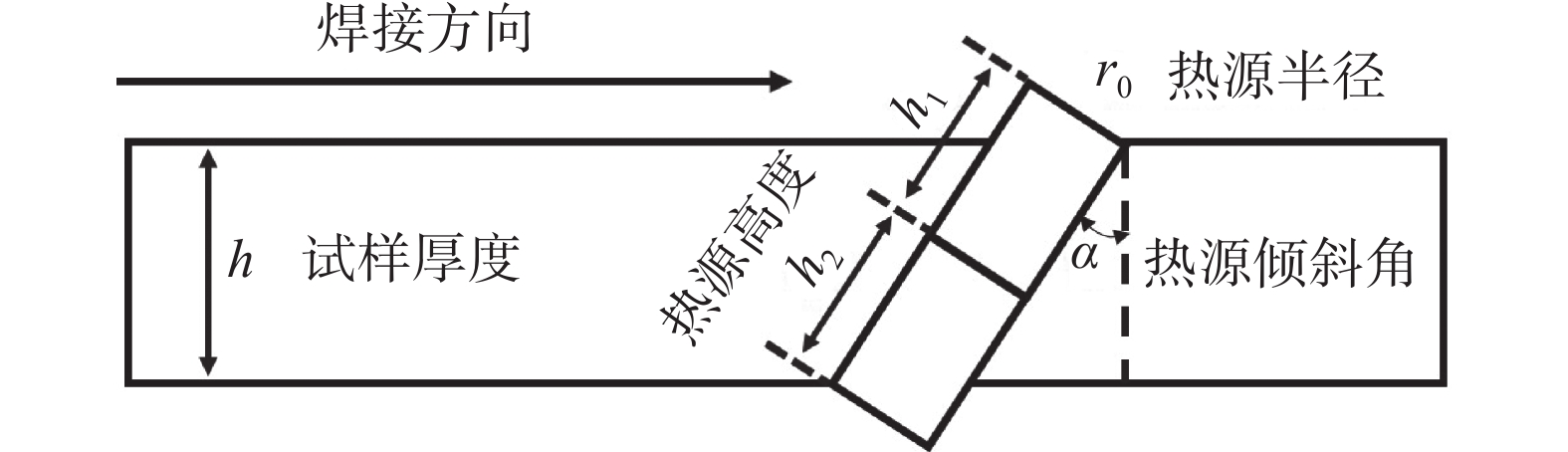
图 5 双柱体倾斜热源模型示意图[11]
Figure 5. Schematic diagram of bipillar heat source parameters
表 1 EH40和316L的化学成分(质量分数,%)
Table 1 Chemical compositions of EH40 and 316L
材料 C Si Mn Cr Ni Mo 其他 Fe EH40 0.12 0.31 1.31 0.04 0.02 — 0.088 余量 316L 0.015 0.55 1.14 16.8 10.1 2.08 0.075 余量 表 2 优化程序的参数设置
Table 2 Parameter Settings of the optimization program
粒子数量
n粒子维度
d位置可行域
[Xmin, Xmax]速度可行域
[Vmin, Vmax]衰减系数
λ最大迭代次数
m1现有最优解的最大迭代次数
m25 2 [(1, 3)T, (3, 5)T] [−(1, 1)T, (1,1)T] 0.5 30 5 表 3 热源参数优化结果
Table 3 Optimization results of heat source parameters
案例编号 迭代次数 计算时间t/h 热源参数 优化结果/mm 试验结果/mm 最小误差δ(%) $ {f_1} $ $ {f_2} $ 上宽 下宽 腰宽 腰宽位置 上宽 下宽 腰宽 腰宽位置 1 17 43.4 1.57 4.18 3.84 3.47 1.47 4.47 3.88 3.36 1.47 4.57 2.07 2 7 17.9 1.41 4.24 3.84 3.47 1.45 4.52 2.15 3 9 23.0 1.91 4.12 3.84 3.47 1.47 4.37 2.07 4 12 30.6 1.35 4.28 3.85 3.47 1.45 4.44 2.11 5 14 35.7 1.53 4.24 3.83 3.45 1.44 4.45 2.06 6 7 17.9 1.54 4.22 3.83 3.45 1.44 4.48 2.06 7 14 35.7 2.49 3.96 3.86 3.51 1.49 4.49 2.84 8 11 28.1 1.70 4.21 3.84 3.47 1.47 4.43 2.07 9 10 25.5 1.56 4.20 3.84 3.47 1.47 4.47 2.07 10 18 45.9 1.54 4.22 3.84 3.45 1.44 4.49 2.06 表 4 不同缩减比例模型的预测结果
Table 4 Predicted results of different reduced models
模型 计算时间
t/h预测结果/mm 最小误差
δ(%)上宽 下宽 腰宽 1/5缩减模型 0.51 3.84 3.45 1.44 2.06 1/2缩减模型 4.23 3.85 3.47 1.47 2.03 完整模型 28.6 3.85 3.47 1.47 2.03 -
[1] 张建强, 张国栋, 姚兵印, 等. 马氏体/奥氏体异种钢焊接接头Ⅳ型蠕变失效数值模拟[J]. 机械工程学报, 2013, 49(12): 78 − 83. doi: 10.3901/JME.2013.12.078 Zhang Jianqiang, Zhang Guodong, Yao Bingyin, et al. Numerical simulation on the type IV creep failure of dissimilar welded joint between T91 and HR3C heat-resistant steels[J]. Journal of Mechanical Engineering, 2013, 49(12): 78 − 83. doi: 10.3901/JME.2013.12.078
[2] Wang Y, Jiang P, Geng S, et al. Influence of the wrinkle surface structures on the vapor flow and keyhole stability in 20 kW high power laser welding[J]. International Journal of Heat and Mass Transfer, 2022, 193(9): 1 − 12.
[3] 蔡建鹏, 叶延洪, 张彦杰, 等. 坡口形式对Q345/SUS304异种钢对接接头残余应力和变形的影响[J]. 机械工程学报, 2015, 51(10): 56 − 61. Cai Jianpeng, Ye Yanhong, Zhang Yanjie, et al. Study on influences of groove type on welding residual stress and deformation in Q345/SUS304 dissimilar ssteel butt-welded joints[J]. Journal of Mechanical Engineering, 2015, 51(10): 56 − 61.
[4] Rong Y, Chang Y, Xu J, et al. Numerical analysis of welding deformation and residual stress in marine propeller nozzle with hybrid laser-arc girth welds[J]. International Journal of Pressure Vessels and Piping, 2017, 158(12): 51 − 58.
[5] Fu G, Lourenço M I, Duan M, et al. Influence of the welding sequence on residual stress and distortion of fillet welded structures[J]. Marine Structures, 2016, 46: 30 − 55.
[6] Harati E, Karlsson L, Svensson L E, et al. The relative effects of residual stresses and weld toe geometry on fatigue life of weldments[J]. International Journal of Fatigue, 2015, 77(4): 160 − 165.
[7] 王苹, 裴宪军, 钱宏亮, 等. 焊接结构抗疲劳设计新方法与应用[J]. 机械工程学报, 2021, 57(16): 349 − 360. doi: 10.3901/JME.2021.16.349 Wang Ping, Pei Xianjun, Qian Hongliang, et al. Unique fatigue design method of welded structures and application[J]. Journal of Mechanical Engineering, 2021, 57(16): 349 − 360. doi: 10.3901/JME.2021.16.349
[8] 邓德安, 梁伟, 罗宇, 等. 采用热弹塑性有限元方法预测低碳钢钢管焊接变形[J]. 焊接学报, 2006, 27(1): 76 − 80. Deng Dean, Liang Wei, Luo Yu, et al. Prediction of welding distortion in a mild steel pipe by means of thermal-elastic-plastic finite element method[J]. Transactions of the China Welding Institution, 2006, 27(1): 76 − 80.
[9] Zhang C, Li S, Sun J, et al. Controlling angular distortion in high strength low alloy steel thick-plate T-joints[J/OL]. Journal of Materials Processing Technology, 2019, 267(September 2018): 257–267.
[10] Deng D, Murakawa H. Influence of transformation induced plasticity on simulated results of welding residual stress in low temperature transformation steel[J]. Computational Materials Science, 2013, 78(6): 55 − 62.
[11] Rong Y, Wang L, Wu R, et al. Visualization and simulation of 1700MS sheet laser welding based on three-dimensional geometries of weld pool and keyhole[J]. International Journal of Thermal Sciences, 2022, 171(9): 1 − 11.
[12] Rong Y, Mi G, Xu J, et al. Laser penetration welding of ship steel EH36: A new heat source and application to predict residual stress considering martensite phase transformation[J]. Marine Structures, 2018, 61: 256 − 267.
[13] Rahman Chukkan J, Vasudevan M, Muthukumaran S, et al. Simulation of laser butt welding of AISI 316L stainless steel sheet using various heat sources and experimental validation[J]. Journal of Materials Processing Technology, 2015, 219(8): 48 − 59.
[14] 杨建国, 陈绪辉, 张学秋. 高能束焊接数值模拟可变新型热源模型的建立[J]. 焊接学报, 2010, 31(2): 25 − 28. Yang Jianguo, Chen Xuhui, Zhang Xueqiu. Numerical modeling of new alterable heat source based on high energy welding beam[J]. Transactions of the China Welding Institution, 2010, 31(2): 25 − 28.
[15] 吴甦, 赵海燕, 王煜, 等. 高能束焊接数值模拟中的新型热源模型[J]. 焊接学报, 2004, 25(1): 91 − 94. Wu Su, Zhao Haiyan, Wang Yu, et al. A new heat source model in numerical simulation of high energy beam welding[J]. Transactions of the China Welding Institution, 2004, 25(1): 91 − 94.
[16] 胥国祥. 激光 + GMAW-P复合热源焊焊缝成形的数值模拟[D]. 山东: 山东大学, 2009. Xu Guoxiang. Numerical simulation of weld formation in laser + GMAW-P hybrid welding [D]. Shandong: Sandong University, 2009.
[17] 张维明, 武传松, 秦国梁, 等. 铝合金激光 + 脉冲GMAW复合焊焊缝成形的预测[J]. 机械工程学报, 2013, 49(10): 110 − 115. doi: 10.3901/JME.2013.10.110 Zhang Weiming, Wu Chuansong, Qin Guoliang, et al. Prediction of Weld Shape and Size for Laser + GMAW-P Hybrid Welding of Aluminium Alloys[J]. Journal of Mechanical Engineering, 2013, 49(10): 110 − 115. doi: 10.3901/JME.2013.10.110
[18] Deng D, Liang W, Murakawa H. Determination of welding deformation in fillet-welded joint by means of numerical simulation and comparison with experimental measurements[J]. Journal of Materials Processing Technology, 2007, 183(2-3): 219 − 225. doi: 10.1016/j.jmatprotec.2006.10.013
[19] Wang L, Zhang G, Xu J, et al. Effect of collapse and hump on thermomechanical behavior in high-power laser welding of 16-mm marine steel EH40[J]. The international Journal of Advanced Manufacturing Technology, 2022, 120(3): 2003 − 2013.
[20] Rong Y, Xu J, Lei T, et al. Microstructure and alloy element distribution of dissimilar joint 316L and EH36 in laser welding[J]. Science and Technology of Welding and Joining, 2018, 23(6): 454 − 461. doi: 10.1080/13621718.2017.1410342
[21] Zhang L, Zhang G, Bai X, et al. Effect of the process parameters on the three-dimensional shape of molten pool during full-penetration laser welding process.[J] The International Journal of Advanced Manufacturing Technology, 2016, 86(5): 1273–1286.
[22] Brickstad B, Josefson B L. A parametric study of residual stresses in multi-pass butt-welded stainless steel pipes[J]. International Journal of Pressure Vessels and Piping, 1998, 75(1): 11 − 25. doi: 10.1016/S0308-0161(97)00117-8
[23] Kennedy J, Eberhart R. Particle swarm optimization[C]//Proceedings of ICNN’95 - International Conference on Neural Networks.preceedings conference on neural netnork. IEEE,1995,4:1942-1948
[24] Rong Y, Xu J. Forming mechanism of weld ccross section and validating thermal aanalysis results based on the maximal temperature field for laser welding[J]. Metals, 2022, 12(774): 1 − 9.
[25] Farias R M, Teixeira P R F, Vilarinho L O. An efficient computational approach for heat source optimization in numerical simulations of arc welding processes[J]. Journal of Constructional Steel Research, 2021, 176(10): 1 − 13.




 下载:
下载: