Process optimization of projection welding of nut based on regression analysis
-
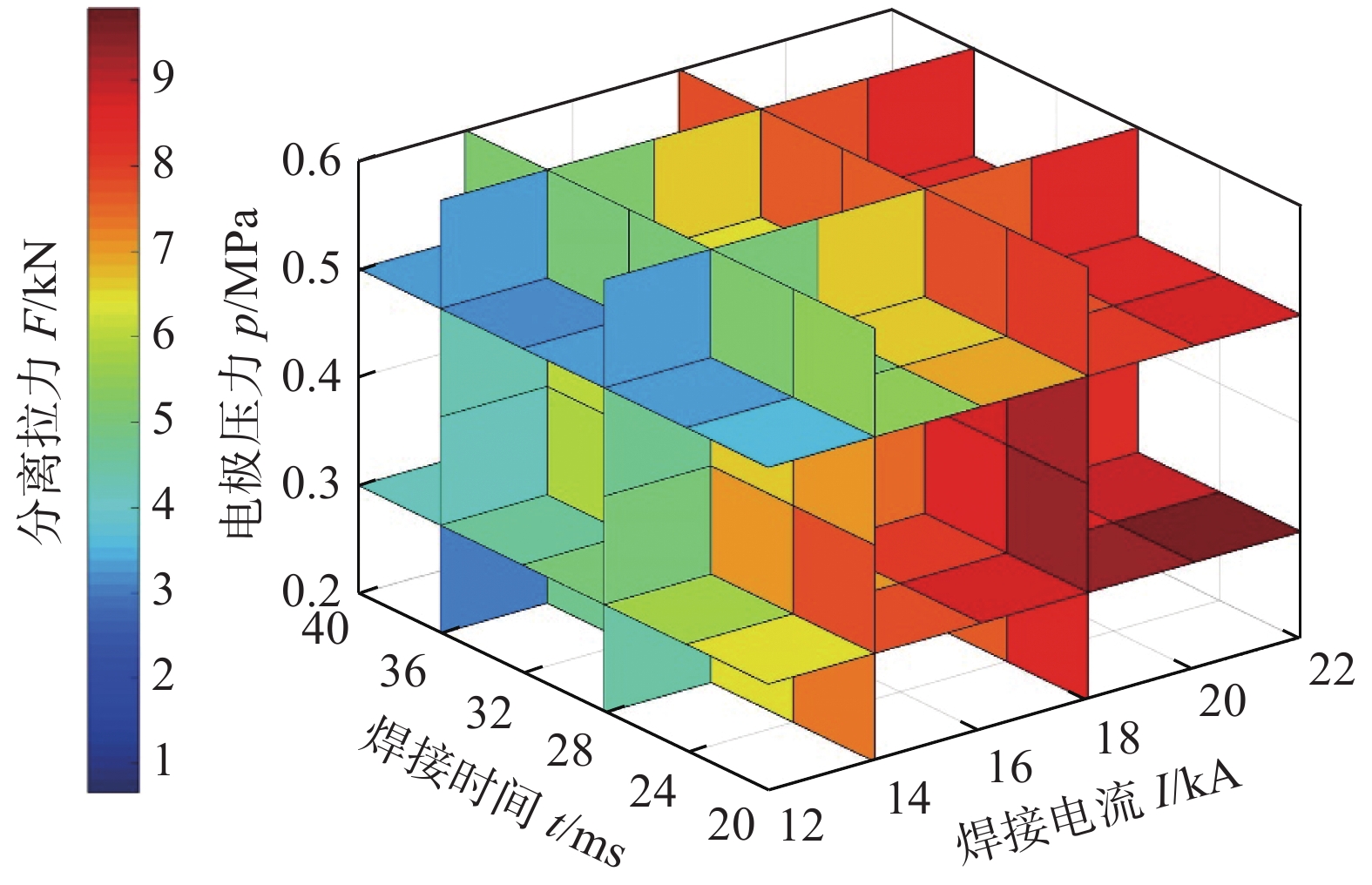
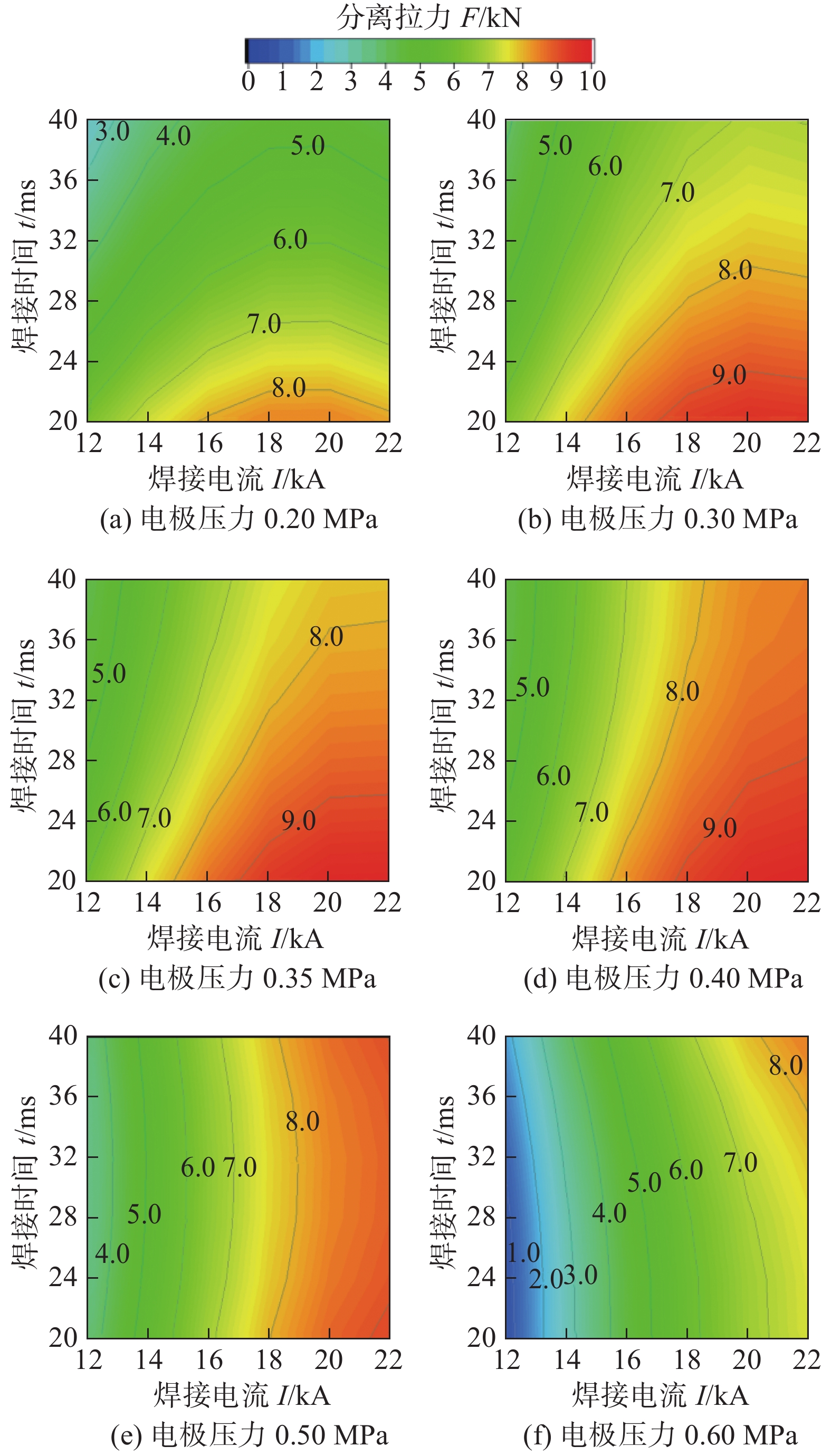
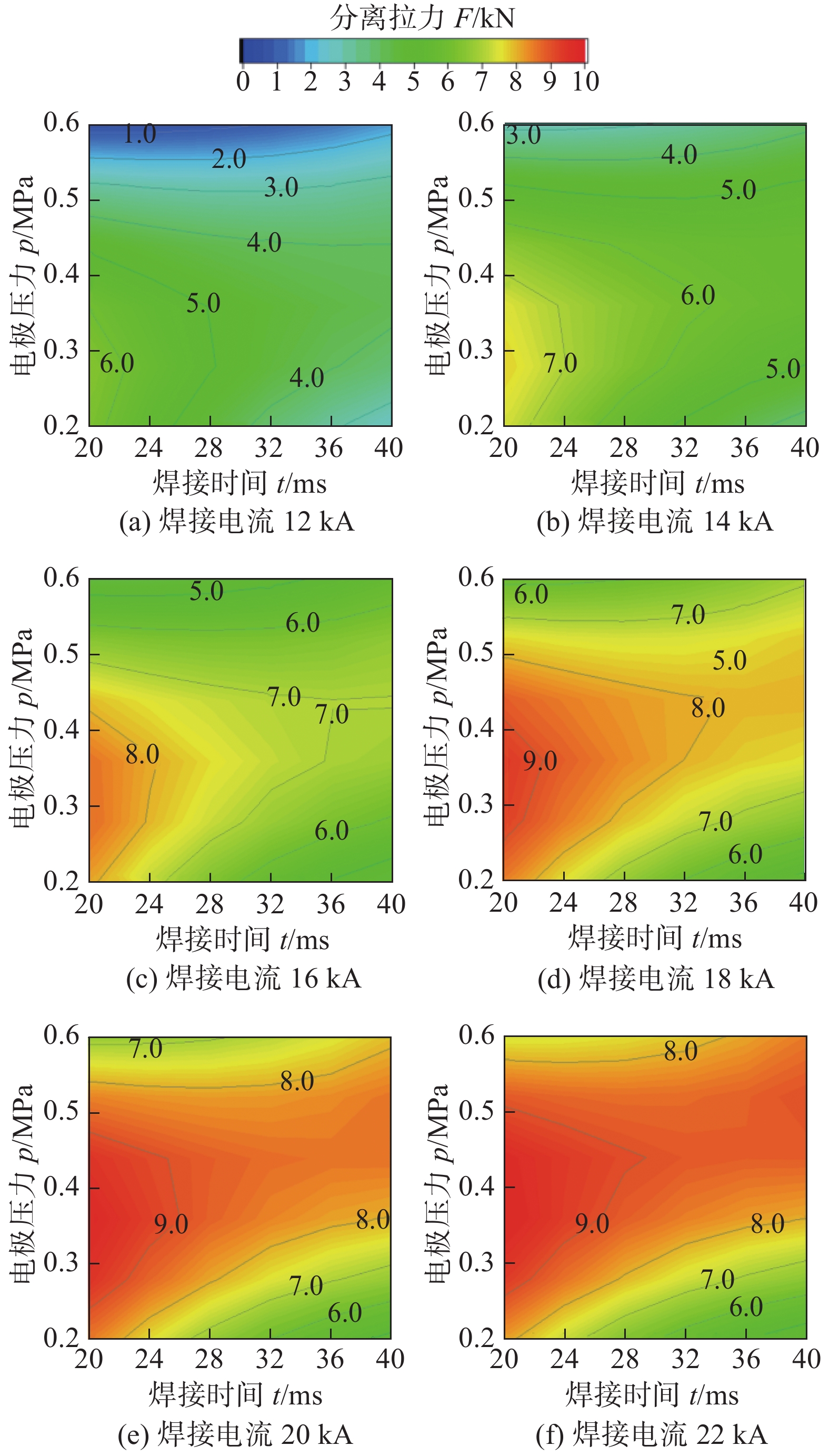
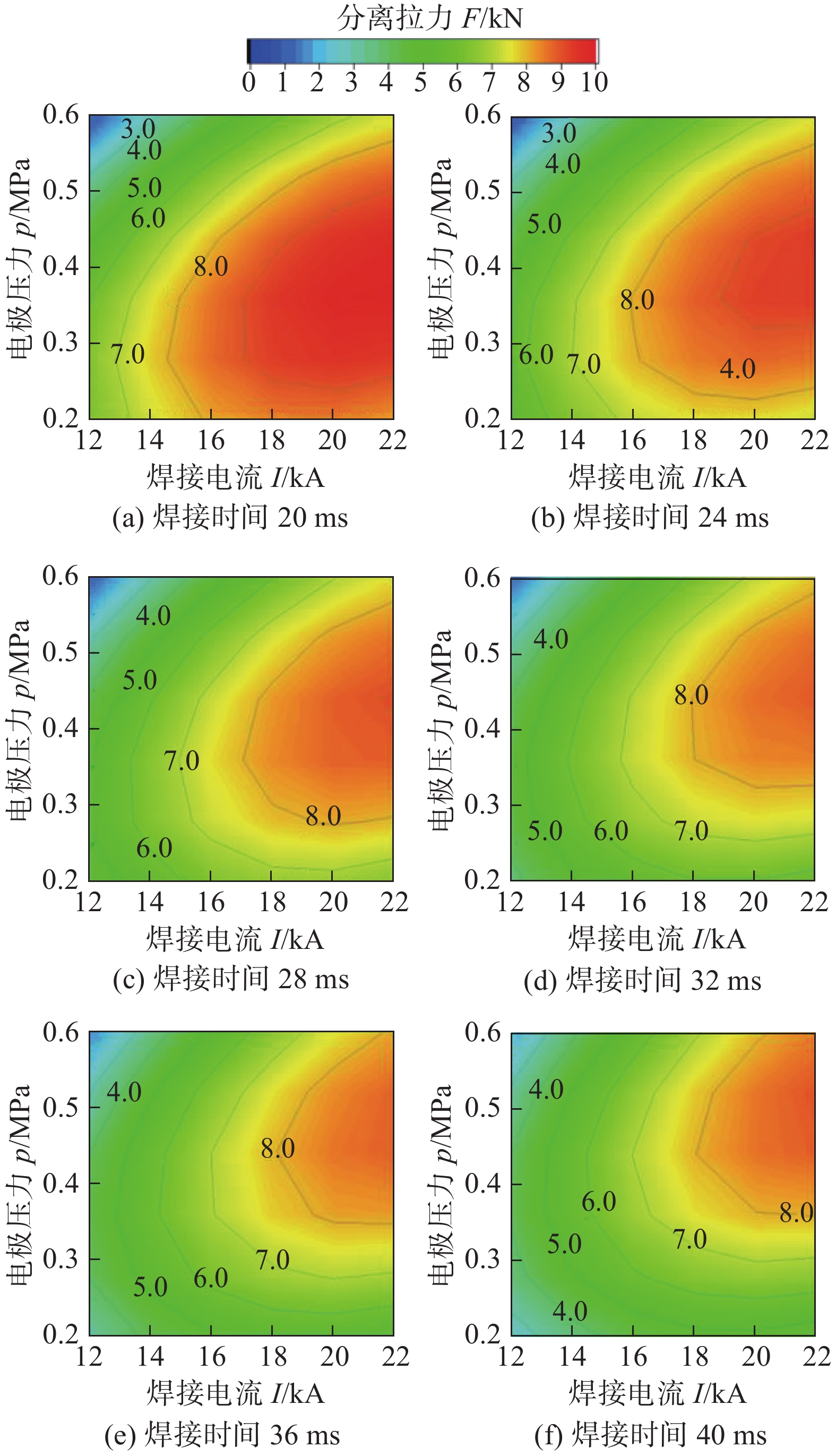
摘要: 通过单因素试验对M6焊接方螺母和SAPH370酸洗热轧钢板进行了焊接，对所焊试样进行分离拉力试验，得出了不同焊接参数下的分离拉力. 基于Spss和Matlab软件对试验数据进行了回归处理，获得了以分离拉力为主要考察指标的焊接质量回归模型. 结果表明，焊接电流对分离拉力的影响最大，焊接时间和电极压力对分离拉力影响较小. 电极压力较小时，焊接时间对分离拉力影响较大；电极压力较大时，焊接电流对分离拉力影响较大. 电极压力和焊接电流的交互作用最大，焊接电流和焊接时间的交互作用以及电极压力和焊接时间的交互作用较小.Abstract: Single factor experimental design was used for M6 welded square nuts and SAPH370 pickled hot-rolled steel plates in order to optimize the nut welded joint quality. The electrode force, welding current and welding time were picked out as the process parameters and the Pull-out load were weighted into a welding quality index. The mathematical model between the welding quality index and process parameters was obtained by regression analysis. The research results show that the welding current has the greatest effect on the Pull-out load, and the welding time and electrode pressure have a small effect on the Pull-out load. When the electrode pressure is small, the welding time has a large effect on the Pull-out load. When the electrode pressure is large, the welding current has a large effect on the Pull-out load. The interaction effect between electrode pressure and welding current is the largest, the interaction effect between welding current and welding time and the interaction effect between electrode pressure and welding time are smaller.
-
Keywords:
- projection welding of nut /
- SAPH370 /
- the pull-out load /
- regression model /
- welding quality
-
0. 序言
焊接结构疲劳评估的主S-N曲线法自提出以来，取得了很好的应用效果，该方法采用与外载荷平衡的结构应力作为参数，解决了焊接结构高周疲劳寿命预测时S-N曲线的选取困难和有限元计算时的网格敏感性问题[1-4]. 2007年针对低周疲劳问题，在ASME BPVC VIII-2标准中给出了伪弹性算法，即将疲劳试验获得的加载—位移曲线外推插值，深入的研究表明该算法是有局限性的，为解决该局限性提出了结构应变法，并推导了弯曲为主和膜应力为主的理想弹塑性材料的结构应变理论解[5]. 产品设计时要求应力低于屈服强度，故而很少存在低周疲劳问题，导致结构应变方法的应用较少[6-9].
2019年，高一迪等人[10]在国内率先在开展了等效结构应变法在低周疲劳范围的适用性研究，结果表明作为结构应力法的延伸，结构应变法可以应用到低周疲劳范围. 近年来，有些铁路货车产品出现了低周疲劳故障问题，线路测试时发现，原来认为弹性状态的构件，在运用过程中确实存在高于屈服的应力响应. 在设计前端，随着轻量化设计要求的提出，一方面结构要减重，另一方面更要充分发挥构件的潜力，所以设计也开始考虑低周疲劳设计[11]. 因此详细梳理结构应变法理论，建立分析流程，开展工程领域的适用性研究是目前亟待开展的研究工作.
鉴于此，在前期成果的基础上，推导理想弹塑性模型下结构应变计算公式，并基于理论算法开展程序的设计和验证. 基于平面应变的有限元模型和结构应变理论解进行理论的适用性分析，提出结构应变法的适用范围，实现低周疲劳的结构应变法在铁路货车上的应用，为该方法在工程领域的推广应用奠定基础.
1. 结构应变法理论
结构应变法的理论假设为：对于完全弹塑性各向同性材料，通过弹性和塑性变形后，假定沿着厚度方向上的变形仍然维持在1个平面上；其次，结构应变法理论是以平面应变状态进行考虑，焊接接头模型如图1所示，采用六面体单元进行网格划分，平板承受拉伸载荷，则焊接接头中部为平面应变状态，两侧为平面应力状态，故可将三维问题简化为平面问题，如按最危险部位考核的话，实际疲劳评估应考虑为平面应变状态.
1.1 结构应变的计算方法
将焊接接头的承载情况分为两种形式：(a)弯曲载荷较大情况，弯曲应力导致板上、下面都发生屈服；(b)膜力载荷较大情况，膜应力导致上表面发生屈服.
1.1.1 上下表面都发生屈服
对于贯穿厚度的1个截面，首先使用弹性状态的结构应力计算的方式，得到膜应力
$ {\sigma _{\text{m}}} $ 和弯曲应力$ {\sigma _{\text{b}}} $ ，两者求和后显然已经超过了屈服强度$ {S_{\text{Y}}} $ ，如图2所示.由于塑性变形原因，弹性轴发生偏移，偏移距离为
$ e $ ，弹性芯变为$ 2c $ ，内、外表面的应变分别为$ {\varepsilon _{\text{0}}} $ 和$ {\varepsilon _{\text{i}}} $ ，内外表面应变对应的结构应力分别为$ {\sigma '_{\text{O}}} $ 和$ {\sigma '_{\text{i}}} $ . 根据弹性状态和塑性状态下的力和弯矩等效，列出$$ \left\{\begin{array}{l}{\displaystyle \sum F=0,\begin{array}{cc}& {\sigma }_{\text{m}}t={S}_{\text{Y}}\left(\dfrac{t}{2}-(c-e)\right)-{S}_{\text{Y}}\left(\dfrac{t}{2}-(c + e)\right)\end{array}}\\ {\displaystyle \sum {M}_{\text{O}}=0,\begin{array}{cc}& \dfrac{{t}^{2}{\sigma }_{\text{b}}}{6}={S}_{\text{Y}}\left(\dfrac{t}{2}-(c-e)\right)\times \dfrac{1}{2}\left(\dfrac{t}{2} + (c-e)\right) + \dfrac{(2c{)}^{2}{S}_{\text{Y}}}{6} + {S}_{\text{Y}}\left(\dfrac{t}{2}-(c + e)\right)\times \dfrac{1}{2}\left(\dfrac{t}{2} + (c + e)\right)\end{array}}\end{array}\right. $$ (1) 式中：
$ {\sigma _{\text{m}}} $ 和$ {\sigma _{\text{b}}} $ 为弹性状态下计算的膜应力和弯曲应力；$ {S_{\text{Y}}} $ 为材料的屈服强度；$ P $ 为拉伸载荷，$ M $ 为弯曲载荷；$ t $ 为板厚；$ e $ 为弹性轴偏离中性层的距离；$ c $ 为发生塑性变形后的中性层与塑性区距离；$ {\varepsilon _{\text{i}}} $ 和$ {\varepsilon _{\text{0}}} $ 为内、外表面的应变；$ {\sigma '_{\text{O}}} $ 和$ {\sigma '_{\text{i}}} $ 为产生内、外表面$ {\varepsilon _{\text{i}}} $ 和$ {\varepsilon _{\text{0}}} $ 时的弹性结构应力.求解后可获得式(2)中的弹性轴偏移距离和弹性芯$$ \left\{ \begin{gathered} e = \dfrac{{{\sigma _{\text{m}}}t}}{{2{S_{\rm{Y}}}}} \\ 2c = t\sqrt {3\left[ {1 - {{\left( {\dfrac{{{\sigma _{\text{m}}}}}{{{S_{\text{Y}}}}}} \right)}^2} - \dfrac{2}{3}\dfrac{{{\sigma _{\text{b}}}}}{{{S_{\text{Y}}}}}} \right]} \\ \end{gathered} \right. $$ (2) 上述计算适用于线性硬化材料或幂硬化材料，弹性芯参数
$ 2c $ 是产生塑性变形后留下的弹性芯大小，中性轴移轴参数$ e $ 是产生塑性变形以后中性轴的偏移量. 在弹性芯参数$ 2c $ 和中性轴移轴公式$ e $ 的计算公式中包含的到$ {\sigma _{\text{m}}} $ 和$ {\sigma _{\text{b}}} $ ，两者需要通过数值分析计算得到.假设弹性核的存在占主导地位，根据材料力学的几何关系，弯曲变形的曲率为$$ \dfrac{1}{R} = \dfrac{M}{{ZI}} = \dfrac{{\dfrac{1}{2}{S_{\text{Y}}} \times c \times \dfrac{2}{3}c \times 2}}{{Z \times \dfrac{{{{(2c)}^3}}}{{12}}}} = \dfrac{{\dfrac{2}{3}{c^2}{S_{\text{Y}}}}}{{\dfrac{2}{3}Z{c^3}}} = \dfrac{{{S_{\text{Y}}}}}{{Zc}} $$ (3) 式中：
$ R $ 为弯曲的曲率半径；$ Z $ 为弹性模量；$ I $ 为惯性矩.发生塑性变性后，中性轴偏移后曲率半径也跟着变化，如图3所示.
根据图3中的变形关系，可以计算出最外层的应变
$ {\varepsilon _{\text{0}}} $ 和最内层的应变$ {\varepsilon _{\text{i}}} $ ，即$$ \begin{gathered} \dfrac{R}{{R + e + \dfrac{t}{2}}} = \dfrac{1}{{1 + 1 \times {\varepsilon _{\text{o}}}}} \Rightarrow {\varepsilon _{\text{o}}} = \dfrac{1}{R}\left(e + \dfrac{t}{2}\right) \\ \dfrac{{\dfrac{t}{2} - e}}{R} = \dfrac{{1 - (1 + {\varepsilon _{\text{i}}})}}{1} \Rightarrow {\varepsilon _{\text{i}}} = \dfrac{1}{R}\left(e - \dfrac{t}{2}\right) \\ \end{gathered} $$ (4) 再根据最外层应变和最内层应变计算膜应变和弯曲应变，即
$$ \begin{gathered} {\varepsilon _{\text{m}}} = \dfrac{{{\varepsilon _{\text{o}}} + {\varepsilon _{\text{i}}}}}{2} = \dfrac{e}{R} \\ {\varepsilon _{\text{b}}} = \dfrac{{{\varepsilon _{\text{o}}} - {\varepsilon _{\text{i}}}}}{2} = \dfrac{t}{{2R}} \\ \end{gathered} $$ (5) 式中：
$ {\varepsilon _{\text{m}}} $ 为膜应变；$ {\varepsilon _{\text{b}}} $ 为弯曲应变.获得膜应变
$ {\varepsilon _{\text{m}}} $ 和弯曲应变$ {\varepsilon _{\text{b}}} $ 后，将两者求和获得结构应变$ {\varepsilon _{\text{s}}} $ ，同等效结构应力计算方法一样，在循环载荷作用下，等效结构应变的参数为$$ \Delta {E_{\text{S}}} = \dfrac{{\Delta {\varepsilon _{\text{S}}}}}{{{t^{\tfrac{{2 - m}}{{2m}}}}I{{(r)}^{\tfrac{1}{m}}}}} $$ (6) 式中：
$ \Delta {E_{\text{s}}} $ 等效结构应变的变化范围；$ \Delta {\varepsilon _{\text{s}}} $ 为结构应变的变化范围；$ m $ 为应力强度因子的幂指数，取值为3.6；$ r $ 为弯曲应变$ {\varepsilon _{\text{b}}} $ 与结构应变$ {\varepsilon _{\text{s}}} $ 的比值；$ I(r)^{\tfrac{1}{n}} $ 为载荷比$ r $ 的无量纲函数[3].等效结构应变计算后，结合主
$ E - N $ 曲线，如表1所示，进行寿命$ N $ 计算，即表 1 主E-N曲线Table 1. Main E-N curve统计依据 试验常数 $ C $ 试验常数 $ h $ 中值 0.10868 0.3195 $ + 2\sigma $ 0.13025 ${{ - } }2\sigma$ 0.06313 $ + 3\sigma $ 0.15610 ${{ - } }3\sigma$ 0.05268 $$ \Delta {E_{\text{S}}} = C \times {N^{ - h}} $$ (7) 式中：
$ C $ 和$ h $ 为试验常数；$ N $ 为寿命.1.1.2 上表面发生屈服
当膜应力较大时，弯曲应力和膜应力只能使板的一侧发生屈服. 对于贯穿厚度的一个截面，使用弹性计算的方式，将得到
$ {\sigma _{\text{m}}} $ 和$ {\sigma _{\text{b}}} $ ，两者求和后只有外表面超过了屈服强度$ {S_{\text{Y}}} $ ，如图4所示.![]()
图 4 上表面发生屈服示意图Figure 4. Schematic diagram of yield on the upper surface relation of deformation根据弹性状态和塑性状态下的力和弯矩等效，得出
$$ \left\{\begin{array}{l}{\displaystyle \sum F=0,\begin{array}{cc}& {\sigma }_{\text{m}}t={S}_{\text{Y}}\left(\dfrac{t}{2}-(c-e)\right)\end{array}}\\ {\displaystyle \sum {M}_{\text{O}}=0,\begin{array}{cc}& \dfrac{{t}^{2}{\sigma }_{\text{b}}}{6}={S}_{\text{Y}}\left(\dfrac{t}{2}-(c-e)\right)\times \dfrac{1}{2}\left(\dfrac{t}{2} + (c\text{ + }e)\right) + \dfrac{(2c{)}^{2}{S}_{\text{Y}}}{6}\end{array}}\end{array}\right. $$ (8) 经计算后，获得中性轴和弹性核，即
$$ \begin{split} & e = \dfrac{1}{8}\dfrac{{{S_{\text{Y}}}t{{\left( { - 3{\sigma _{\text{m}}} - {\sigma _{\text{b}}} + 3{S_{\text{Y}}}} \right)}^2}}}{{{{\left( { - {\sigma _{\text{m}}} + {S_{\text{Y}}}} \right)}^3}}} - \dfrac{1}{2}\dfrac{{\left( { - 2{\sigma _{\text{m}}} - {\sigma _{\text{b}}} + 2{S_{\text{Y}}}} \right)t}}{{ - {\sigma _{\text{m}}} + {S_{\text{Y}}}}} \\& c = \dfrac{t}{2}\left( {\dfrac{{ - 3{\sigma _{\text{m}}} - {\sigma _{\text{b}}} + 3{S_{\text{Y}}}}}{{ - {\sigma _{\text{m}}} + {S_{\text{Y}}}}}} \right) \end{split} $$ (9) 根据材料力学的几何关系，弯曲变形的曲率为
$$ \dfrac{1}{R} = \dfrac{{8{{({S_{\text{Y}}} - {\sigma _{\text{m}}})}^3}}}{{tE{{(3{S_{\text{Y}}} - 3{\sigma _{\text{m}}} - {\sigma _{\text{b}}})}^2}}} $$ (10) 根据曲率公式，计算外表面和内表面的应变为
$$ \begin{gathered} {\varepsilon _{\text{o}}} = \dfrac{{{S_{\text{Y}}}}}{E} + \dfrac{{(t - c)}}{R} \\ {\varepsilon _{\text{i}}} = \dfrac{{{S_{\text{Y}}}}}{E} - \dfrac{c}{R} \\ \end{gathered} $$ (11) 膜应变和弯曲应变、等效结构应变计算公式与式(5)、式(6)一致，寿命计算如式(7)所示. 用有效的屈服应力
$ {S'_{\text{Y}}} $ 替换上述公式中材料的屈服强度$ {S_{\text{Y}}} $ 为$$ {S'_{\text{Y}}} = \dfrac{{{S_{\text{Y}}}}}{{\sqrt {1 - \nu + {\nu ^2}} }} $$ (12) 式中：
$ \nu $ 为泊松比.式(12)将上述公式扩展到平面应变问题. 如果使用冯米塞斯准则，可用下式的
$ E' $ 替换$ E $ .$$ E' = \dfrac{E}{{1 - {\nu ^2}}} $$ (13) 1.1.3 结构应变法的计算实施流程
在弯曲为主的加载式(1)中，将弯矩等效整理后为
$$ {\sigma _{\text{b}}} = \dfrac{{6{S_{\text{Y}}}}}{{{t^2}}}\left[\dfrac{{{t^2}}}{4} - \dfrac{{{c^2}}}{3} - \dfrac{{\sigma _{\text{m}}^2{t^2}}}{{4S_{\text{Y}}^2}}\right]\left( {0 \leqslant c \leqslant \dfrac{t}{2} - e} \right) $$ (14) 如图2所示，当
$ c = 0 $ 时，整个截面均达到塑性，求解式(14)，得$$ {\sigma _{{\text{bmax}}}} = \dfrac{{6{S_{\text{Y}}}}}{{{t^2}}}\left[\dfrac{{{t^2}}}{4} - \dfrac{{\sigma _{\text{m}}^2{t^2}}}{{4S_{\text{Y}}^2}}\right] = \dfrac{3}{2}{S_{\text{Y}}}\left[ {1 - \dfrac{{\sigma _{\text{m}}^2}}{{S_{\text{Y}}^2}}} \right] $$ (15) 如图4所示，当
$ c = 0.5t - e $ 时，仅截面一侧发生塑性变形，求解式(14)，得$$ \begin{split} {\sigma _{{\text{bmin}}}}& = \dfrac{{6{S_{\text{Y}}}}}{{{t^2}}}\left[\dfrac{{{t^2}}}{4} - \dfrac{1}{3}{\left(\dfrac{t}{2} - e\right)^2} - \dfrac{{\sigma _{\text{m}}^2{t^2}}}{{4S_{\text{Y}}^2}}\right]\\& = {S_{\text{Y}}}\left[ {1 + \dfrac{{\sigma _{\text{m}}^{}}}{{S_{\text{Y}}^{}}} - \dfrac{{2\sigma _{\text{m}}^{\text{2}}}}{{S_{\text{Y}}^2}}} \right] \end{split}$$ (16) 根据以上计算结果，以弹性计算获得结构应力
$ {\sigma _{\text{s}}} = {\sigma _{\text{m}}} + {\sigma _{\text{b}}} $ 后，整个截面存在以上几种状态：(1)当$ {\sigma _{\text{s}}} \leqslant {S_{\text{Y}}} $ 时，截面没有发生屈服；(2)当$ {\sigma _{\text{b}}} \leqslant {\sigma _{{\text{bmin}}}} $ ，且$ {\sigma _{\text{s}}}> {S_{\text{Y}}} $ 时，外表面都发生屈服；(3)当$ {\sigma _{{\text{bmin}}}} \leqslant {\sigma _{\text{b}}} \leqslant {\sigma _{{\text{bmax}}}} $ ，且$ {\sigma _{\text{s}}} > {S_{\text{Y}}} $ 时，内、外表面都发生屈服；(4)当$ {\sigma _{\text{b}}} > > {\sigma _{{\text{bmax}}}} $ 时，截面发生塑性失效. 基于上述原理程序流程如图5所示.![]()
图 5 焊接结构高、低周疲劳实施流程Figure 5. Calculation process of high and low cycle fatigue of welded structures2. 试验验证及分析
2.1 试验验证
以Q450NQR1材料的搭接接头试样为研究对象，焊接接头尺寸示意图如图6所示，为保证试验的准确性，使用能够实现塑性应变测量的测试胶水进行应变片粘贴.应变片布置如图7所示，在距离焊趾C截面2 mm、17 mm的B截面、A截面位置的上下表面布置应变片.
![]()
图 6 焊接接头宽板试样示意图(mm)Figure 6. Schematic diagram of wide plate specimen of welded joint值得注意的是由于搭接接头产生了附加弯矩，搭接接头长度为300 mm，两端夹具的夹持长度分别为80 mm，在仿真模型上一定要考虑夹持长度. 另外，由于板的宽度和应变片尺寸限制，导致布置的测点并不在1条直线上，但由于是小试样，不会产生较大影响. 弹性加载下的应变测试结果如表2所示，由于构件焊接过程中存在焊接变形，首次加载后，构件会发生1次变形调整，卸载后再重新加载，测试结果稳定，如图8所示.根据表2中的应变测试结果，采用结构应力的方法进行应力集中系数计算. 第1次拉伸时，试验存在变形微校正，选择拉伸第2次的数据计算应力集中系数为
表 2 弹性加载下的应变测试Table 2. strain test under elastic loading载荷类型 加载次数n/次 载荷值P/kN 实测应变 ε(10−6) 1号 2号 3号 4号 5号 6号 拉伸 1 25 714 251 1342 −14 1593 45 2 25 771 198 802 112 814 122 3 25 771 197 785 115 811 123 压缩 1 10 330 −61 −320 −37 −336 −35 2 25 −859 −140 −818 −86 −852 −87 3 25 −803 −163 −790 −100 −820 −107 ![]()
图 8 焊接接头应变片布置及应变测试Figure 8. Strain gauge arrangement and strain testing of welded joints$$ \left\{\begin{gathered} {S_1} = 158.8\;\;{\text{MPa}}，\begin{array}{*{20}{c}} {}&{{S_2} = 40.8\;\;{\text{MPa}}} \end{array} \\ {S_3} = 165.2\;\;{\text{MPa}}，\begin{array}{*{20}{c}} {}&{{S_4} = 23.0\;\;{\text{MPa}}} \end{array} \\ \end{gathered}\right. $$ (17) $$ \left\{\begin{gathered} \sigma _{\text{b}}^{\text{B}} = \dfrac{1}{2}({S_1} - {S_2}) = 59.0\;\;{\text{MPa}} \\ \sigma _{\text{m}}^{\text{B}} = \dfrac{1}{2}({S_1} + {S_2}) = 99.8\;\;{\text{MPa}} \\ \sigma _{\text{b}}^{\text{A}} = \dfrac{1}{2}({S_3} - {S_4}) = 71.1\;\;{\text{MPa}} \\ \end{gathered}\right. $$ (18) $$\begin{split} \sigma _{\text{s}}^{\text{C}}& = \sigma _{\text{m}}^{\text{C}} + \sigma _{\text{b}}^{\text{C}} = \sigma _{\text{m}}^{\text{A}} + \sigma _{\text{b}}^{\text{A}} + \dfrac{{17}}{{15}}(\sigma _{\text{b}}^{\text{B}} - \sigma _{\text{b}}^{\text{A}})\\& { = {S_1}} + \dfrac{{17}}{{15}}(\sigma _{\text{b}}^{\text{B}} - \sigma _{\text{b}}^{\text{A}}) \\ &{ = 158.8 + } \dfrac{{17}}{{15}}(71.1 - 59.0)\\&{ = 172.54\;\;{\text{MPa}}} \end{split} $$ (19) 通过
$ \sigma _{\text{s}}^{\text{C}} $ 和名义应力比值(25 kN下的名义应力为100 MPa)计算出结构应力集中系数为1.73. 通过试验机采用正弦曲线将试样加载到95 kN载荷(名义应力为380 MPa)，实测的应变数据如图9所示.按实测的应力集中系数1.73，380 MPa载荷下弯矩引起的最大应力为273.6 MPa. 根据上表面屈服的结构应变的式(8) ~ 式(11)可得：上表面焊趾的微应变为2910，焊趾下表面为460. 膜应变和弯曲应变分别为1685和1225. 弹性模量E为206000，
$ m $ 为3.6，板厚$ t $ 为5 mm. 则弯曲比$ r $ ，载荷控制下$I{(r)^{\tfrac{1}{m}}} $ 和等效结构应变$ \Delta {E_{\text{s}}} $ 分别为$$ r = \dfrac{{{\varepsilon _{\text{b}}}}}{{{\varepsilon _{\text{s}}}}} = \dfrac{{1\;225}}{{2\;910}} = 0.420\;9 $$ (20) $$ I{(r)^{\tfrac{1}{m}}} = \dfrac{{1.23 - 0.364r - 0.17{r^2}}}{{1.007 - 0.306r - 0.178{r^2}}} = 1.236\;2 $$ (21) $$ \Delta {E_s} = \dfrac{{\Delta {\varepsilon _s}}}{{{t^{\tfrac{{2 - m}}{{2m}}}}I{{(r)}^{\tfrac{1}{m}}}}} = \dfrac{{2\;910}}{{0.699\;3 \times 1.236\;2}} = 3\;366 $$ (22) 根据式(7)计算了该试样在不同统计值下的寿命，并与疲劳试验结果进行了对比，结果如表3所示. 可见，中值下的低周疲劳寿命计算结果与试验值吻合.
表 3 寿命计算Table 3. Life calculation寿命计算值(次) 寿命试验值
(次)中值 ( + 2σ) (−2σ) ( + 3σ) (−3σ) 34780 149984 8065 646779 1870 24201 2.2 适用性分析
建立的平面应变有限元模型如图10所示，模型一端施加对称约束，另一端施加膜应力和弯曲应力载荷，在靠近对称约束位置，建立路径，路径的起点和终点如图10所示.
理论算法和平面应变的有限元模型对比如图11所示. 固定端的膜应力载荷
$ {\sigma _{\text{m}}} $ 取为250 MPa，300 MPa，350 MPa和380 MPa，通过改变端部弯曲应力载荷，计算不同弯曲应力载荷下平面应变模型和结构应变理论解对比如图11(a) ~ 图11(d)所示.![]()
图 11 理论算法和平面应变的有限元模型对比Figure 11. Comparison between theoretical algorithm and plane strain finite element model. (a) the membrane stress is 250 MPa; (b) the membrane stress is 300 MPa; (c) the membrane stress is 350 MPa; (d) the membrane stress is 380 MPa; (e) the bending stress is 250 MPa; (f) the bending stress is 325 MPa固定端的弯曲应力载荷
$ {\sigma _b} $ 取250 MPa、325 MPa，通过改变端部膜应力载荷，计算不同膜应力载荷. 平面应变模型和结构应变理论解对比如图11(e)、图11(f)所示.计算结果表明：当材料的屈服为550 MPa，当弯曲载荷与膜应力载荷合载荷为700 MPa时，平面应变模型和结构应变理论解结果完全一致，高于700 MPa后，随着载荷的增加，误差也随着增加.3. 应用实例
以货车车体为研究对象，采用板壳单元建模，单元长度为30 mm，将车体考虑为柔性体，采用固定交界面法完成柔性体计算 [12-13]. 为了能够施加与实际状态更为吻合的载荷，以试验台模型作为车体仿真的边界条件[14]. 货车虚拟试验模型如图12所示.在虚拟试验台的车钩加载端，施加制动等效压缩载荷，载荷达到峰值后的车体的最大主应力云图如图13所示，主应力最大的位置集中在车体底盘，在车钩和两侧过度位置主应力集中较为严重.一般来讲，主应力与结构正应力往往有正相关的关系，因此我们根据车体最大主应力云图对关心焊缝进行筛选. 提取了如图13所示的A1和B1位置的正应力，发现A1位置明显超过Q450NQR1钢的屈服极限，B1位置与屈服极限接近，如图14所示.通过计算的节点力先计算结构应力，再根据2.1节中的计算流程计算出结构应变，对比了结构应力法和结构应变的损伤对比结果，如图15所示.
![]()
图 13 变形及最大主应力云图Figure 13. Cloud map of deformation and maximum principal stress. (a) first side of car body; (b) second side of car body![]()
图 15 基于结构应力和结构应变所得损伤对比Figure 15. Comparison of damage based on structural stress and structural strain. (a) The position of A1; (b) The position of B1A1位置 ~ A4位置由于结构正应力超出材料比例极限较多，因此塑性变形比较明显，这导致了基于结构应变计算的车体疲劳损伤超过基于结构应力计算的车体疲劳损伤，以A1位置为例，基于结构应变计算的车体疲劳损伤比基于结构应力计算的车体疲劳损伤高2倍.而在车钩附近的B1位置 ~ B4位置，由于结构正应力超出材料屈服强度不多，因此基于结构应变计算得到疲劳损伤仅仅略大于基于结构应力计算得到的疲劳损伤.虽然近几年针对低周疲劳问题，针对更为精确的材料应力应变性能曲线，提出了更为准确的计算方法，但由于理想弹塑性材料不需要材料的力学性能曲线，所以开展的结构应变法在工程具有很大的应用价值.
4. 结论
(1)为了解决铁路货车的低周疲劳评估问题，详细梳理低周疲劳的结构应变法理论，完成了程序设计和方法的适用性验证.
(2)平面应变有限元模型和结构应变法理论解的对比结果表明，基于理想弹性性模型的结构应变方法及程序计算的Q450NQR1材料，当结构应力高出屈服强度150 MPa以内时，平面应变模型和结构应变理论解结果完全一致，超过该范围后，随着载荷的增加，误差也增加.
(3)低周疲劳的结构应变法现阶段能够满足铁路货车的设计需求，研究为该方法的工程推广应用提供了良好的技术支撑.
-
![]()
图 4 不同电极压力下分离拉力随焊接参数变化二维图
Figure 4. Two-dimensional plot of the pull-out load at different electrode pressures. (a) P = 0.20 MPa; (b) P = 0.30 MPa; (c) P = 0.35 MPa; (d) P = 0.40 MPa; (e) P = 0.50 MPa; (f) P = 0.60 MPa
![]()
图 5 不同焊接电流下分离拉力随焊接参数变化二维图
Figure 5. Two-dimensional plot of the pull-out load at different welding currents. (a) I = 12 kA; (b) I = 14 kA; (c) I = 16 kA; (d) I = 18 kA; (e) I = 20 kA; (f) I = 22 kA
![]()
图 6 不同焊接时间下分离拉力随焊接参数变化二维图
Figure 6. Two-dimensional plot of the pull-out load at different welding times. (a) t = 20 ms; (b) t = 24 ms; (c) t = 28 ms; (d) t = 32 ms; (e) t = 36 ms; (f) t = 40 ms
表 1 螺母化学成分(质量分数，%)
Table 1 Chemical composition of nut
C Si Mn P S Fe 0.13 ~ 0.18 ≤ 0.08 0.30 ~ 0.60 ≤ 0.025 ≤ 0.015 余量 
下载: 导出CSV
表 2 板材化学成分(质量分数，%)
Table 2 Chemical composition of steel sheet
C Si Mn P S Fe ≤ 0.21 ≤ 0.30 ≤ 0.75 ≤ 0.035 ≤ 0.035 余量
下载: 导出CSV
表 3 板材力学性能
Table 3 Mechanical properties of steel sheet
厚度
δ/mm抗拉强度
Rm/MPa上屈服强度
ReH/MPa断后伸长率
A(%)1.5 ≥ 370 ≥ 225 ≥ 32
下载: 导出CSV
表 4 单因素试验参数
Table 4 Single factor test parameters
焊接参数 电极压力
p/MPa焊接电流
I/kA焊接时间
t/ms水平1 0.2 12 20 水平2 0.3 14 24 水平3 0.35 16 28 水平4 0.4 18 32 水平5 0.5 20 36 水平6 0.6 22 40
下载: 导出CSV
表 5 分离拉力试验结果
Table 5 Pull-out load test results
序号 电极压力p/MPa 焊接电流I/kA 焊接时间t/ms 分离拉力
实际值F/kN分离拉力
预测值$ \tilde F $ /kN序号 电极压力p/MPa 焊接电流I/kA 焊接时间t/ms 分离拉力
实际值F/kN分离拉力
预测值$ \tilde F $ /kN1 0.5 12 36 3.3 3.31 10 0.4 20 36 7.4 8.44 2 0.5 14 36 4.85 5.14 11 0.5 20 36 6 8.47 3 0.5 16 36 9.3 6.61 12 0.6 20 36 7.85 7.47 4 0.5 18 36 7.9 7.72 13 0.5 20 20 8.85 8.75 5 0.5 20 36 9.75 8.47 14 0.5 20 24 9.6 8.54 6 0.5 22 36 10.65 8.85 15 0.5 20 28 7.6 8.42 7 0.2 20 36 5.2 5.31 16 0.5 20 32 7.5 8.39 8 0.3 20 36 9 7.39 17 0.5 20 36 10.85 8.47 9 0.35 20 36 8.55 8.04 18 0.5 20 40 8 8.63
下载: 导出CSV
表 6 回归系数
Table 6 Coefficients of the equation
a0 a1 a2 a3 a12 a13 a23 a11 a22 a33 0.26 0.274 1.479 −0.49 1.21 0.608 0 −51.211 −0.045 0.003
下载: 导出CSV
-
[1] Tolf E, Hedegård J. Resistance nut welding: improving the weldability and joint properties of ultra high strength steels[J]. Welding in the World, 2007, 51(3−4): 28 − 36. doi: 10.1007/BF03266557
[2] Nielsen C V, Zhang W, Martins P A F, et al. Numerical and experimental analysis of resistance projection welding of square nuts to sheets[J]. Procedia Engineering, 2014, 81: 2141 − 2146. doi: 10.1016/j.proeng.2014.10.299
[3] Cao X, Zhou Z, Luo J, et al. Capacitor discharge welding of nuts to steel sheets[J]. Journal of Materials Processing Technology, 2019, 264: 486 − 493. doi: 10.1016/j.jmatprotec.2018.09.038
[4] Wang Xiaopei, Zhang Yongqiang. Effects of welding procedures on resistance projection welding of nuts to sheets[J]. ISIJ International, 2017, 57(12): 2194 − 2200. doi: 10.2355/isijinternational.ISIJINT-2017-219
[5] MiKNo, Zygmunt, et al. Analysis of resistance welding processes and expulsion of liquid metal from the weld nugget[J]. Archives of Civil and Mechanical Engineering, 2018, 18(2): 522 − 531. doi: 10.1016/j.acme.2017.08.003
[6] 赵大伟, 梁东杰, 王元勋, 等. 基于回归分析的钛合金微电阻点焊焊接工艺优化[J]. 焊接学报, 2018, 39(4): 79 − 83. doi: 10.12073/j.hjxb.2018390100 Zhao Dawei, Liang Dongjie, Wang Yuanxun, et al. Research on process parameters optimization of small scale resistance spot welding via regression analysis[J]. Transactions of the China Welding Institution, 2018, 39(4): 79 − 83. doi: 10.12073/j.hjxb.2018390100
[7] 曹小兵, 周照耀, 邹春华, 等. 高强度钢储能螺母凸焊工艺非线性多元回归设计[J]. 焊接, 2018(1): 34 − 37. Cao Xiaobing, Zhou Zhaoyao, Zhou Zhaoyao, et al. Nonlinear multi-variate regression design of capacitor discharge welding of high strength steel nut[J]. Welding & Joining, 2018(1): 34 − 37.
[8] 赵大伟, 梁东杰, 王元勋, 等. 基于田口法的钛合金微电阻点焊焊接参数优化[J]. 焊接学报, 2018, 39(5): 101 − 104. doi: 10.12073/j.hjxb.2018390132 Zhao Dawei, Liang Dongjie, Wang Yuanxun, et al. Parameters optimization of small scale spot welding for titanium alloy via Taguchi experiment and grey relational analysis[J]. Transactions of the China Welding Institution, 2018, 39(5): 101 − 104. doi: 10.12073/j.hjxb.2018390132
[9] 赵大伟, 康与云, 易荣涛, 等. 基于试验设计与统计分析的双相钢激光焊工艺优化[J]. 焊接学报, 2018, 39(1): 65 − 69. doi: 10.12073/j.hjxb.2018390015 Zhao Dawei, Kang Yuyun, Yi Rongtao, et al. Research on process parameters optimization of laser welding for dual phase steel[J]. Transactions of the China Welding Institution, 2018, 39(1): 65 − 69. doi: 10.12073/j.hjxb.2018390015
[10] Zhao D, Wang Y, Liang D, et al. Modeling and process analysis of resistance spot welded DP600 joints based on regression analysis[J]. Materials & Design, 2016, 110(2): 676 − 684.
[11] Zhao D, Wang Y, Liang D, et al. Performances of regression model and artificial neural network in monitoring welding quality based on power signal[J]. Journal of Materials Research and Technology, 2019, 9: 1231 − 1240.
[12] Zhao X, Wang C, Zhang R, et al. Parameter selecting and quality predicting of spot welding based on artificial neural networks[J]. China Welding, 1998, 7(2): 4 − 8.
-
期刊类型引用(2)
1. 李传胜，王雷，郭糠，杨祥帆，于文晶，王利忠，王洪宝，张怀强，张英波. Mo对钛合金激光焊接接头组织性能影响研究. 电焊机. 2025(01): 73-79 . 
百度学术
2. 李海英，熊淑秋. 船用耐蚀材料激光焊接技术分析. 舰船科学技术. 2024(21): 67-70 . 百度学术
其他类型引用(0)
计量
- 文章访问数: 603
- HTML全文浏览量: 75
- PDF下载量: 28
- 被引次数: 2
