Microstructure and mechanical properties of joints of a new Al-Mg-Mn-Er alloy by TIG welding
-
摘要: 对一种Al-Mg-Mn-Er合金薄板进行TIG填丝焊接，并研究接头的微观组织以及力学性能. 结果表明，焊缝中心为等轴树枝晶，熔合线附近未出现典型的联生结晶形貌，而是存在着一个宽度约为100 μm的细晶带，热影响区出现再结晶组织. 焊缝中的析出相主要以初生Al3Er的形式存在，与母材相比，焊缝中初生Al3Er的尺寸更加细小，分布更加均匀，焊缝中次生Al3Er的数量相对较少，而且这些次生Al3Er是焊接时母材中未熔化而保留下来的. 焊接区和热影响区的硬度均低于母材，其中焊缝区的硬度最低. 随着焊接热输入的增加，接头的抗拉强度先增加后减小，当焊接热输入为218 J/mm时，接头的抗拉强度最高，达到母材的71.4%，试样的断裂位置均位于焊缝区，断口形貌呈现韧性断裂特征.
-
关键词:
- Al-Mg-Mn-Er合金 /
- TIG焊 /
- 微观组织 /
- 力学性能
Abstract: New Al-Mg-Mn-Er alloy sheets were welded by TIG welding with filler wires. The microstructure and mechanical properties of the joints were studied. The results show that the center of the weld seam is mainly composed of equiaxed crystals. There is no typical epitaxial solidification appeared, but a fine-grained zone with width of 100 μm is formed near the fusion line. The recrystallized structure is appeared in the heat affected zone. The precipitates in the weld zone mainly exist in the form of primary Al3Er. Compared with the base metal, the size of primary Al3Er in the weld seam is smaller and its distribution is more uniform. The number of secondary Al3Er in the weld seam is relatively small and the secondary Al3Er is from the base metal. The microhardness of the weld zone and the heat affected zone are lower than that of base metal, and the microhardness of the weld zone is the lowest. With the increase of the welding heat input, the tensile strength of the joints increases first and then decreases. When the welding heat input is 218 J/mm, the tensile strength of the joint is the highest which is 71.4% of that of the base metal. The fracture of all tensile samples is located at the weld zone, and the fracture morphology shows the typical ductile fracture characteristics.-
Keywords:
- Al-Mg-Mn-Er alloy /
- TIG welding /
- microstructure /
- mechanical properties
-
0. 序言
Al-Mg合金具有重量轻、塑性好、耐腐蚀、焊接性良好等特点，被广泛应用在船舶、军工、航空航天、交通运输等领域. 随着新技术、新材料的不断出现，对Al-Mg合金的力学性能也提出了更高要求. 微合金化是改善Al-Mg合金力学性能的重要途径，钪(Sc)是研究最深入、强化效果最显著的微合金化元素[1]，然而Sc的价格非常昂贵，其使用受到限制. 研究表明[2]，铒(Er)具有与Sc元素类似的作用，可以有效细化铸态组织，改善力学性能和耐腐蚀性能，而且铒的价格远低于钪，因此含铒铝合金正逐步成为一类具有广阔应用前景和发展空间的新型稀土铝合金.
任何先进材料的广泛使用，不仅依赖于自身的优异性能，也取决于相应成形技术的进步. 焊接作为铝合金的一种重要连接手段，具有快速加热、极速冷却的特点，与铸造工艺明显不同，在焊接热循环的作用下，接头的微观组织与母材存在较大差异，接头的力学性能也会随之改变，容易出现软化、疲劳强度降低等现象[3]，因此随着铝合金综合性能的改善以及应用领域的扩大，铝合金焊接技术已经成为世界范围内焊接领域的研究热点. 然而目前新型含铒铝合金的研究主要集中在合金的成分、组织及性能等方面，关于含铒铝合金熔化焊接头微观组织与性能的研究还处于起始阶段，Er元素在接头中的存在形式和作用机制尚不明确.
近年来为了提高铝合金焊接接头的质量，激光焊[4]、电子束焊[5]等新技术得到了一定程度的应用，但受到设备成本、场地条件等因素的限制，不适合在工业生产中大规模推广. 钨极惰性气体保护焊(TIG焊)具有成本低、操作灵活、焊接质量好、工艺过程稳定等优点，被广泛应用于Al-Mg合金的焊接. 因此对一种Al-Mg-Mn-Er合金薄板进行TIG填丝焊接，研究接头的微观组织及力学性能，为这种新型合金在焊接结构上的应用提供理论依据.
1. 试验方法
试验母材为1.5 mm厚的冷轧态板材，其化学成分为(质量分数，%)：4.5% Mg， 0.7% Mn，0.4% Er，其余为Al. 从母材上截取规格为1.5 mm × 1.5 mm的焊丝作为TIG焊填充材料. 焊接前对母材和焊丝的表面进行化学处理，以去除油污及氧化膜，然后将母材固定在夹具上进行焊接，焊道垂直于母材轧制方向. 试验选用WSE-300型钨极氩弧焊电源，电源类型为交流，采用氩气保护，氩气气体流量为15 L/min，采用5组不同的焊接热输入进行施焊，具体参数见表1 (热效率按80%计算).
表 1 TIG焊工艺参数Table 1. TIG welding parameters编号 焊接电流
I/A焊接电压
U/V焊接速度
v/(mm·s−1)热输入
E/(J·mm−1)1 75 16.5 5 198 2 80 17.0 5 218 3 85 17.6 5 239 4 90 18.2 5 262 5 100 19.1 5 306 检查焊缝无气孔、裂纹等缺陷后，垂直于焊道方向切取金相试样. 试样经过磨光、抛光、Kerell试剂腐蚀处理后，在DMI5000M型金相显微镜上进行分析. 在QUANTA200型扫描电镜(SEM)上观察微观形貌，并使用SEM自带的能谱仪(EDS)分析微区成分. 采用JEM-2010型透射电镜(TEM)分析薄膜样品的精细结构. 试样抛光后在HXD-1000型维氏硬度计上测量横截面的硬度分布，试验载荷0.98 N，加载时间15 s. 使用XRF-1800型X射线荧光光谱仪(XRF)检测母材以及焊缝金属的化学成分. 按照国家标准的要求制作母材和焊接接头的拉伸试样，在MTS-810型电子万能试验机上完成室温拉伸试验，取3组有效数据的平均值，拉断后的试样用S-3400N型扫描电镜(SEM)观察断口形貌.
2. 试验结果与分析
2.1 焊接接头的微观组织
图1为2号试样的金相组织.图1a ~ 1d分别为试样焊缝中心、熔合区、热影响区以及母材的金相组织. 由图1a可知，焊缝中心主要为等轴树枝晶，但尺寸上有所区别，较大的等轴晶呈现雪花状，尺寸在20 ~ 30 μm之间. 焊缝的结晶形态主要由冷却条件及焊缝金属成分决定. 焊缝的凝固过程与铸造类似，通常都是由边界向心部推进. 焊缝金属的凝固一般起始于熔池边界，由于熔池的边界区域具有较大的温度梯度和较小的结晶速度，因而有利于柱状晶的形成；随着凝固区域逐渐远离熔池边界，熔体中的温度梯度逐渐减小，结晶速度逐渐增大，溶质浓度逐渐增加，致使成分过冷程度不断增大，因此焊缝中心容易形成等轴晶. 此外，母材中添加的稀土元素Er容易与Al元素发生反应，生成难熔的Al3Er[6]. Al3Er为面心立方结构，其晶格常数(0.422 nm)与Al的晶格常数(0.405 nm)十分接近，错配度仅为3.8%，符合成为异质形核核心的结构以及尺寸条件[7]，使得Al3Er在结晶过程中能够促进异质形核，有利于等轴晶的产生，同时也起到细化晶粒的作用.
![]()
图 1 2号试样的金相组织Figure 1. Optical micrographs of welded joint of No.2 sample. (a) weld center; (b) transition zone; (c) HAZ; (d) base metal图1b为熔合区附近的金相组织. 熔合线附近未出现典型的联生结晶形貌，而是存在着一个特殊的等轴细晶带，宽度约为100 μm. Kostrivas等人[8]在2195铝锂合金TIG焊接头的熔合区中也发现了细晶带，并且认为这种细晶带的形成是一个形核、长大的过程，而非再结晶的结果. 根据传统的凝固理论，等轴晶区的形成需满足两个条件，即较高的过冷度和较多的异质形核颗粒. 经过分析，文中熔合区出现等轴细晶带的主要原因是：首先，熔池内部存在着剧烈的对流，使得溶质在熔体中的分布更加均匀，但未熔化的热影响区显然处于静止状态，因此固液界面处必然存在一个流动速度从零到熔体本身流动速度的过渡区. 该过渡区受熔池的搅拌作用较小，流动速度很低且流动方向平行于固液界面，所以在固液界面法线方向不可能发生对流传质，溶质只能通过缓慢的扩散方式传输到对流的熔体中，结果在过渡区中形成了溶质的聚集，增加了成分过冷，为等轴细晶带的产生创造了条件. 其次，过渡区以母材成分为主，而母材中存在大量Al3Er粒子，由于Al3Er的熔点(约1 067 ℃)远高于基体的熔点，基本不发生回熔，能够作为异质形核的核心，有效提高形核率，促进细晶带的形成. 综上可以认为，细晶带的出现是熔池边界的过冷度条件以及异质颗粒的共同作用.图1c为热影响区，纤维状组织已经不明显，原有轧制组织受焊接热循环的作用而发生了一定程度的再结晶. 远离焊缝的母材仍保持典型的冷轧态纤维状组织(见图1d).
2.2 焊缝中的析出相
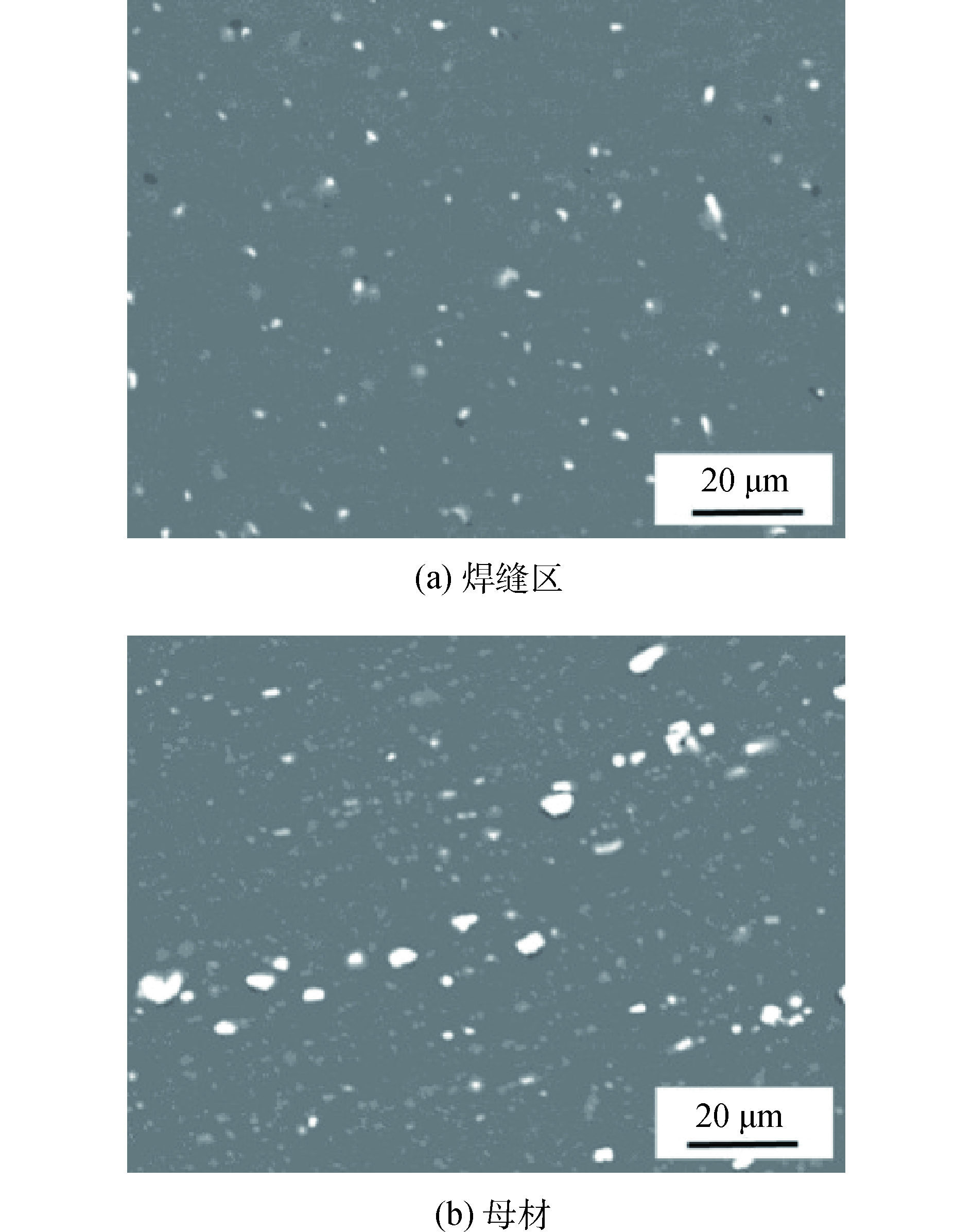
图2为2号试样焊缝区与母材BSE形貌.由焊缝区背散射电子形貌(图2a)可见，焊缝区存在着大量形状不规则的白色析出相，尺寸约为1 ~ 3 μm. 对析出相进行能谱分析，析出相的具体成分为（质量分数，%）：71.23% Al(质量分数，下同)，20.62% Er，2.05% Mg，6.10% Mn. 通常认为[9]，在非平衡条件下，当铝合金中Mn含量小于1%时，Mn基本上均固溶在基体中，当Mn含量大于1%时，会析出Al6Mn. 而母材中的Mn含量仅为0.7%，所以焊缝中的Mn元素主要固溶在Al基体中，很难形成Al6Mn. 基于上述分析，并结合文献[10]可以判断该析出相为初生Al3Er. 在平衡条件下，Er元素在Al原子中的最大固溶度为0.05%，Al-Er合金共晶成分点的Er元素含量约为6%[11]. 母材中Er元素含量远低于6%，在平衡凝固条件下很难形成Al3Er元素. 但是在实际的结晶过程中，焊接熔池中总是存在着能量起伏、结构起伏以及成分起伏. 由于Er的原子半径(1.76 Å)远大于Al(1.43 Å)，只有Er原子在大角度晶界处偏聚，才能有效降低体系的自由能[12]，因此随着Er元素在晶界处的不断富集，当Er元素含量达到共晶或过共晶成分时将会析出初生Al3Er.
![]()
图 2 2号试样焊缝区与母材BSE形貌Figure 2. BSE iamges of weld zone and base metal of No.2 sample. (a) weld seam; (b) base metal对比2号试样焊缝区(图2a)和母材(图2b)背散射电子形貌可以看出，焊缝中初生Al3Er的尺寸更加细小，分布更加均匀. 焊缝中析出相的细化与焊接熔池的结晶特点有关. 焊接熔池体积小，冷却速度快，因此凝固时间较短，从液相中析出的Al3Er没有足够时间长大，使得焊缝中Al3Er的尺寸较母材更加细小. 析出相分布的均匀化则与熔池的流动有关. 在浮力、表面张力以及等离子流力等驱动力的作用下，焊接熔池会产生明显的流动，致使第二相粒子的分布更加均匀.
采用透射电镜观察2号试样焊缝区的精细结构，结果见图3. 可以看出，焊缝区存在着一定数量且相互缠绕的位错线，在位错线附近分布有颗粒状析出相，尺寸约为10 ~ 20 nm，如图3箭头所示. 析出相的具体成分为(质量分数，%)：85.23% Al，12.62% Er，0.65% Mg，1.50% Mn. 结合文献[10]可以推断，这些纳米级析出相为次生Al3Er. 一般情况下，铸造态含铒铝合金需要均匀化处理或时效处理才能获得纳米级次生Al3Er[13]. 焊接与铸造具有类似的凝固过程，并且焊缝熔池的冷却速率远大于铸造，致使液态熔池中次生相的析出更加困难，因此在焊缝金属中很难直接析出次生Al3Er. 基于上述分析可以判断，焊缝金属中观察到的次生Al3Er粒子并非在熔池凝固过程中形成的，而是从原来母材中保留下来的. 由于Al3Er的熔点(约1 067 ℃)远高于基体的熔点，在焊缝金属熔化过程中基本不会发生回熔，因此母材中原有的部分次生Al3Er粒子没有被熔化而被保留在焊缝中. 与母材相比，焊缝中残留的次生Al3Er的数量较少，且很难与再次凝固的焊缝金属继续保持共格关系，其沉淀强化作用十分有限.
![]()
图 3 2号试样焊缝区TEM形貌Figure 3. TEM images of weld zone of No.2 sample. (a) low- magnification image; (b) high-magnification image2.3 焊接接头的力学性能
2.3.1 显微硬度
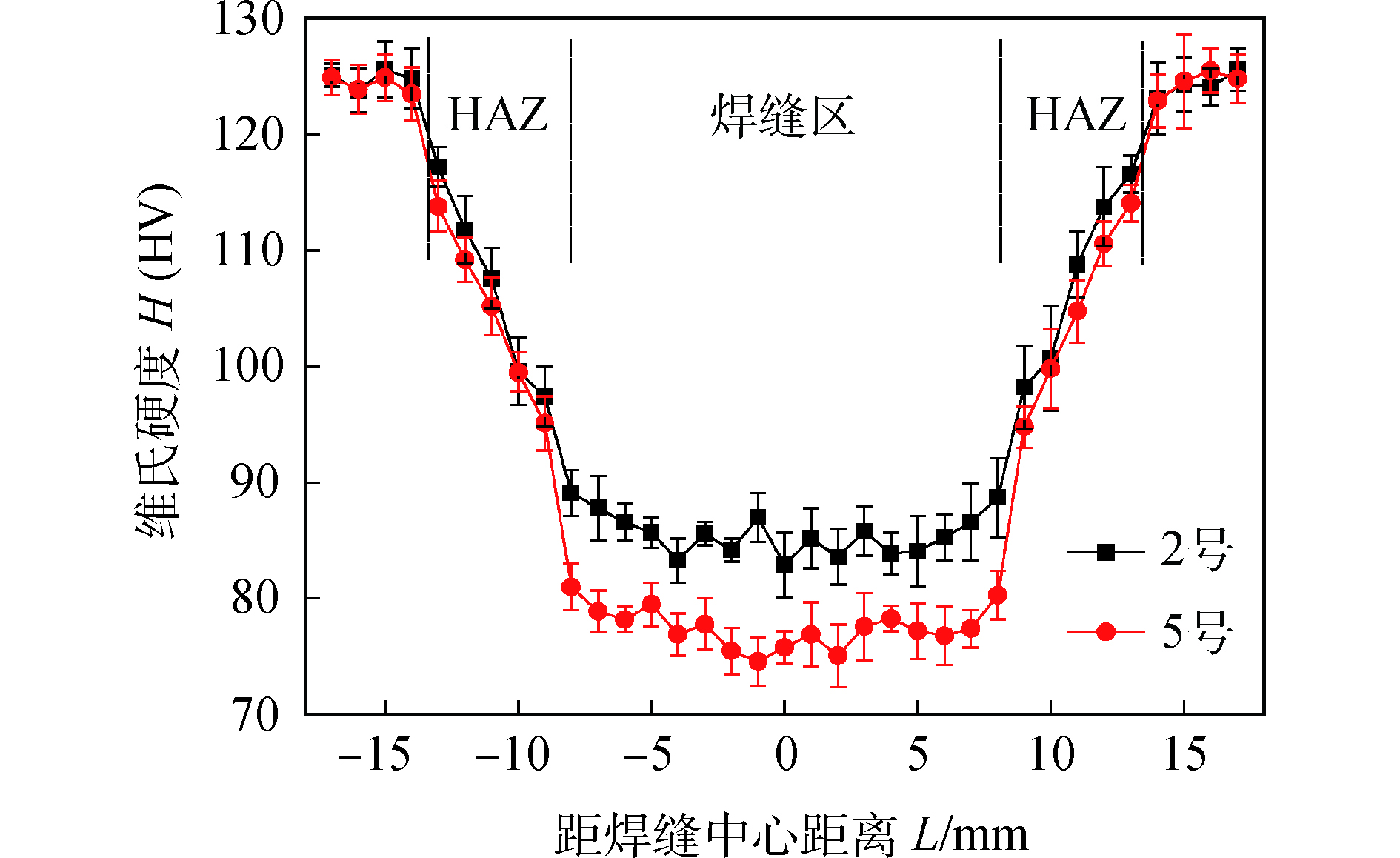
2号和5号接头横截面的显微硬度分布如图4所示. 在焊接过程中，因为热循环的影响，致使焊接接头的不同区域表现出不同的硬度值，又由于铝合金的硬度与强度基本成正比[14]，因此图4也表征了接头强度的分布特征. 由图4可知，两组接头的硬度值以焊缝中间线为中心呈对称分布，焊缝区和热影响区的硬度均低于母材，其中焊缝区的硬度最低，可见焊缝区是整个焊接接头中最薄弱的部分. 其中，2号试样焊缝区平均硬度高于5号试样，这表明2号试样的软化程度相对较轻.
由图4可知，焊接接头的焊缝区和热影响区均存在软化现象. 焊缝区软化的主要原因是：①Al-Mg合金强度的提高一般采用形变强化的方法来实现. 母材在轧制过程中，晶粒产生严重变形，位错密度增加，从而造成硬度以及强度的提高. 在焊接过程中，母材在焊接热循环的作用下熔化并重新凝固，形成类似铸态的焊缝组织，与形变硬化的母材相比，焊缝区的形变强化作用完全消失，导致焊缝区的强度较母材有所下降. ②较高的电弧温度极易造成低熔点金属元素Mg的烧损[15]，使焊缝成分发生变化. 对母材和2号试样焊缝区进行X射线荧光光谱(XRF)检测，结果见表2. 从成分分析结果可以看出，焊缝区的Mg含量与母材相比降低了35%. Mg元素作为Al-Mg合金中的重要强化元素，其含量的下降也在一定程度上降低了焊缝金属的力学性能[16]. ③文献[13, 17]表明，母材经固溶、时效处理后析出的次生Al3Er可以有效阻碍位错运动，起到沉淀强化的作用. 由前文分析可知，焊缝金属中残留的次生Al3Er是从原来母材中保留下来的，并非在焊接过程中直接形成的. 这些次生相数量较少，且很难与再次凝固的焊缝金属继续保持共格关系，母材中原有的沉淀强化作用减弱，造成焊缝区力学性能的降低. 热影响区硬度的下降主要与轧制态母材经历焊接热循环有关. 在焊接过程中，由于焊接热循环的作用使得热影响区附近的轧制组织发生了回复与再结晶，部分或者全部失去由形变硬化产生的强化效果，因而发生软化.
表 2 XRF检测结果(质量分数，%)Table 2. XRF testing results区域 Al Mg Mn Er 母材 94.41 4.58 0.65 0.36 焊缝区 96.10 2.98 0.59 0.33 2.3.2 拉伸性能
在室温条件下，对母材以及5组焊接接头进行静载拉伸试验，结果列于表3. 可以看出，随着焊接热输入的增加，接头的抗拉强度先增加后减小，当焊接热输入为218 J/mm时，接头的抗拉强度最高，达到母材的71.4%. 焊接接头的抗拉强度均低于母材，试样的断裂位置也均位于焊缝区，再次说明焊缝区是整个接头中最薄弱的区域.
表 3 拉伸试验结果Table 3. Tensile test average results序号 热输入E/(J·mm−1) 抗拉强度Rm/MPa 接头系数φ(%) 断裂位置 1 198 297 70.7 焊缝 2 218 300 71.4 焊缝 3 239 292 69.5 焊缝 4 262 288 68.6 焊缝 5 306 285 67.9 焊缝 母材 — 420 — — 2.3.3 断口形貌
图5为2号和5号试样的拉伸断口形貌. 可以看出，拉伸断口均由许多细小的韧窝组成，呈韧性断裂的典型特征. 相对而言，2号试样的韧窝明显更小、更浅，这说明2号试样具有较高的强度和较低的塑性，与拉伸试验结果吻合.在韧窝底部还观察到一些第二相粒子，见图5b和图5d. 对粒子1、粒子2进行能谱分析，粒子1的具体成分为(质量分数，%)：74.23% Al，21.22% Er，1.37% Mg， 3.18% Mn，粒子2的具体成分为：72.48% Al，23.73% Er，1.15% Mg，2.64% Mn. 这些粒子主要由Al，Er两种元素组成，结合前文可推断其为初生Al3Er. 在拉伸过程中，基体首先发生变形，而第二相粒子的塑性较差很难发生变形，无法与基体的变形协同一致，随着整体变形量的增加，基体与第二相粒子之间的变形差异不断增大，容易造成局部应力集中现象，当达到极限时，基体与第二相粒子之间将出现微裂纹，微裂纹在外力的作用下迅速扩展，最终导致接头断裂.
![]()
图 5 拉伸试样断口形貌Figure 5. Fracture morphology of tensile test samples. (a) morpholgy of No.2 sample under low magnification, (b) morpholgy of No.2 sample under high magnification, (c) morpholgy of No.5 sample under low magnification, (d) morpholgy of No.5 sample under high magnification3. 结论
(1)焊缝中心为等轴树枝晶，熔合线附近未出现典型的联生结晶形貌，而是存在着一个宽度约为100 μm的细晶带，热影响区出现再结晶组织.
(2)焊缝中的析出相主要以初生Al3Er的形式存在，与母材相比，焊缝中初生Al3Er的尺寸更加细小，分布更加均匀，焊缝中次生Al3Er的数量相对较少，这些次生Al3Er是焊接时母材中未熔化保留下来的.
(3)焊接接头的显微硬度以焊缝中间线为中心呈对称分布，焊接区和热影响区的硬度均低于母材，其中焊缝区的硬度最低.
(4)随着焊接热输入的增加，接头的抗拉强度先增加后减小，当焊接热输入为218 J/mm时，接头的抗拉强度最高，达到母材的71.4%. 试样的断裂位置均位于焊缝区，拉伸断口由许多细小的韧窝组成，呈韧性断裂的典型特征.
-
![]()
图 1 2号试样的金相组织
Figure 1. Optical micrographs of welded joint of No.2 sample. (a) weld center; (b) transition zone; (c) HAZ; (d) base metal
![]()
图 2 2号试样焊缝区与母材BSE形貌
Figure 2. BSE iamges of weld zone and base metal of No.2 sample. (a) weld seam; (b) base metal
![]()
图 3 2号试样焊缝区TEM形貌
Figure 3. TEM images of weld zone of No.2 sample. (a) low- magnification image; (b) high-magnification image
![]()
图 5 拉伸试样断口形貌
Figure 5. Fracture morphology of tensile test samples. (a) morpholgy of No.2 sample under low magnification, (b) morpholgy of No.2 sample under high magnification, (c) morpholgy of No.5 sample under low magnification, (d) morpholgy of No.5 sample under high magnification
表 1 TIG焊工艺参数
Table 1 TIG welding parameters
编号 焊接电流
I/A焊接电压
U/V焊接速度
v/(mm·s−1)热输入
E/(J·mm−1)1 75 16.5 5 198 2 80 17.0 5 218 3 85 17.6 5 239 4 90 18.2 5 262 5 100 19.1 5 306 
下载: 导出CSV
表 2 XRF检测结果(质量分数，%)
Table 2 XRF testing results
区域 Al Mg Mn Er 母材 94.41 4.58 0.65 0.36 焊缝区 96.10 2.98 0.59 0.33
下载: 导出CSV
表 3 拉伸试验结果
Table 3 Tensile test average results
序号 热输入E/(J·mm−1) 抗拉强度Rm/MPa 接头系数φ(%) 断裂位置 1 198 297 70.7 焊缝 2 218 300 71.4 焊缝 3 239 292 69.5 焊缝 4 262 288 68.6 焊缝 5 306 285 67.9 焊缝 母材 — 420 — —
下载: 导出CSV
-
[1] 周民, 甘培原, 邓鸿华, 等. 含钪微合金化铝合金研究现状及发展趋势[J]. 中国材料进展, 2018, 37(2): 154 − 160. Zhou Min, Gan Peiyuan, Deng Honghua, et al. Research status and prospect of Sc microalloying aluminum alloys[J]. Materials China, 2018, 37(2): 154 − 160.
[2] Liu T H, Wen S P, Wu X L, et al. Effect of trace element Hf on the precipitation process and recrystallization resistance of Al-Er-Zr alloys[J]. Materials Science Forum, 2017, 898: 3 − 8. doi: 10.4028/www.scientific.net/MSF.898.3
[3] Moreira P M G P, Figueiredo M A V D, Castro P M S T D. Fatigue behaviour of FSW and MIG weldments for two aluminium alloys[J]. Theoretical and Applied Fracture Mechanics, 2007, 48(2): 169 − 177. doi: 10.1016/j.tafmec.2007.06.001
[4] Zhou Li, Zhang Mingjun, Jin Xiangzhong, et al. Study on the burning loss of magnesium in fiber laser welding of an Al-Mg alloy by optical emission spectroscopy[J]. International Journal of Advanced Manufacturing Technology, 2017, 88(5-8): 1373 − 1381. doi: 10.1007/s00170-016-8867-y
[5] Jiang Xunyan, Cheng Donghai, Chen Yiping, et al. The superplastic deformation of electron beam welded aluminum lithium alloy[J]. China Welding, 2018, 27(1): 41 − 45.
[6] Li Yonggang, Wei Yinghui, Hou Lifeng, et al. Effect of erbium on microstructures and properties of Mg-Al intermetallic[J]. Journal of Rare Earths, 2014, 32(11): 1064 − 1072. doi: 10.1016/S1002-0721(14)60184-8
[7] Yang Junjun, Nie Zuoren, Jin Tounan, et al. Effect of trace rare earth element Er on high pure Al[J]. Transactions of Nonferrous Metals Society of China, 2003, 13(5): 1035 − 1039.
[8] Kostrivas A, Lippold J C. Fusion boundary microstructure evolution in aluminium alloys[J]. Welding in the World, 2006, 50(11-12): 24 − 34. doi: 10.1007/BF03263458
[9] 王祝堂, 田荣璋.铝合金及其加工手册[M]. 长沙: 中南大学出版社, 1989. [10] Wen S P, Gao K Y, Li Y, et al. Synergetic effect of Er and Zr on the precipitation hardening of Al-Er-Zr alloy[J]. Scripta Materialia, 2011, 65(7): 592 − 595. doi: 10.1016/j.scriptamat.2011.06.033
[11] Mondolfo L F. 铝合金的组织与性能[M]. 王祝堂等译. 北京: 冶金工业出版社, 1988. [12] Mark C C. Improvements to the strength and corrosion resistance of Al-Mg-Mn alloys of near-AA5083 chemistry[D]. Columbus: The Ohio State University, 2001.
[13] Wen S P, Xing Z B, Huang H, et al. The effect of erbium on the microstructure and mechanical properties of Al-Mg-Mn-Zr alloy[J]. Materials Science and Engineering A, 2009, 516(1): 42 − 49.
[14] 温林秀, 赵运强, 董春林, 等. 1561铝合金搅拌摩擦焊接过程压力特征及接头组织性能分析[J]. 焊接学报, 2019, 40(12): 91 − 96. Wen Linxiu, Zhao Yunqiang, Dong Chunlin, et al. Analysis on characteristics of welding pressure,microstructures and mechanical properties of friction stir welded 1561 aluminum alloy[J]. Transactions of the China Welding Institution, 2019, 40(12): 91 − 96.
[15] 陈澄, 薛松柏, 孙乎浩, 等. 5083铝合金TIG焊接头组织与性能分析[J]. 焊接学报, 2016, 44(6): 92 − 97. Chen Cheng, Xue Songbai, Sun Huhao, et al. Microstructure and mechanical properties of 5083 aluminum alloy joint by TIG welding[J]. Transactions of the China Welding Institution, 2016, 44(6): 92 − 97.
[16] 闫德俊, 李海洋, 刘晓莉, 等. 船用5083铝合金变极性等离子焊接头组织和性能[J]. 稀有金属材料与工程, 2018, 47(10): 3161 − 3166. Yan Dejun, Li Haiyang, Liu Xiaoli, et al. Microstructure and mechanical properties of variable polarity plasma arc welded joints of 5083 aluminum alloy for shipbuilding[J]. Rare Metal Material and Engineering, 2018, 47(10): 3161 − 3166.
[17] 聂祚仁, 文胜平, 黄晖, 等. 铒微合金化铝合金的研究进展[J]. 中国有色金属学报, 2011, 21(10): 2361 − 2370. Nie Zuoren, Wen Shengping, Huang Hui, et al. Research progress of Er-containing aluminum alloy[J]. The Chinese Journal of Nonferrous Metals, 2011, 21(10): 2361 − 2370.
-
期刊类型引用(3)
1. 龚利华，郭为民. 紫外光对铝合金焊接接头腐蚀行为的影响. 焊接学报. 2022(04): 106-112+120 . 
本站查看
2. 毛晓东，谷宁杰，宋小雨，任思蒙，路丽英，李虎田. 焊丝成分对5E61铝合金TIG焊接头组织和性能的影响. 焊接学报. 2022(04): 86-93+118-119 . 本站查看
3. 石晓平，王广青，李恒，邢峰. 含Er焊丝对基于PLC控制的激光焊接头的影响. 应用激光. 2022(11): 8-15 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 437
- HTML全文浏览量: 102
- PDF下载量: 4
- 被引次数: 4
